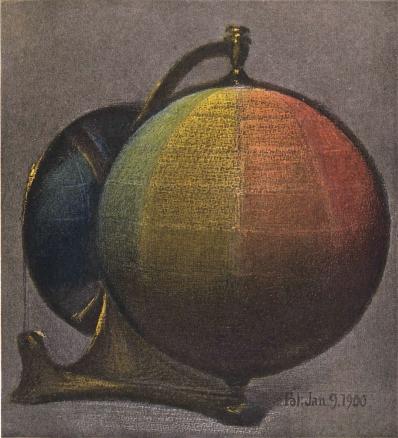The Project Gutenberg EBook of A Color Notation, by Albert H. Munsell

This eBook is for the use of anyone anywhere at no cost and with
almost no restrictions whatsoever.  You may copy it, give it away or
re-use it under the terms of the Project Gutenberg License included
with this eBook or online at www.gutenberg.org


Title: A Color Notation
       A measured color system, based on the three qualities Hue,
              Value and Chroma

Author: Albert H. Munsell

Release Date: July 14, 2008 [EBook #26054]

Language: English

Character set encoding: UTF-8

*** START OF THIS PROJECT GUTENBERG EBOOK A COLOR NOTATION ***




Produced by Louise Hope, K.D. Thornton and the Online
Distributed Proofreading Team at http://www.pgdp.net

9

Chapter I.
COLOR NAMES.

Writing from Samoa to Sidney Colvin in London, Stevenson1 says: “Perhaps in the same way it might amuse you to send us any pattern of wall paper that might strike you as cheap, pretty, and suitable for a room in a hot and extremely bright climate. It should be borne in mind that our climate can be extremely dark, too. Our sitting-room is to be in varnished wood. The room I have particularly in mind is a sort of bed and sitting room, pretty large, lit on three sides, and the colour in favour of its proprietor at present is a topazy yellow. But then with what colour to relieve it? For a little work-room of my own at the back I should rather like to see some patterns of unglossy—well, I’ll be hanged if I can describe this red. It’s not Turkish, and it’s not Roman, and it’s not Indian; but it seems to partake of the last two, and yet it can’t be either of them, because it ought to be able to go with vermilion. Ah, what a tangled web we weave! Anyway, with what brains you have left choose me and send me some—many—patterns of the exact shade.”

(1) Where could be found a more delightful cry for some rational way to describe color? He wants “a topazy yellow” and a red that is not Turkish nor Roman nor Indian, but that “seems to partake of the last two, and yet it can’t be either of them.” As a cap to the climax comes his demand for “patterns of the exact shade.” Thus one of the clearest and most forceful writers of 10 English finds himself unable to describe the color he wants. And why? Simply because popular language does not clearly state a single one of the three qualities united in every color, and which must be known before one may even hope to convey his color conceptions to another.

(2) The incongruous and bizarre nature of our present color names must appear to any thoughtful person. Baby blue, peacock blue, Nile green, apple green, lemon yellow, straw yellow, rose pink, heliotrope, royal purple, Magenta, Solferino, plum, and automobile are popular terms, conveying different ideas to different persons and utterly failing to define colors. The terms used for a single hue, such as pea green, sea green, olive green, grass green, sage green, evergreen, invisible green, are not to be trusted in ordering a piece of cloth. They invite mistakes and disappointment. Not only are they inaccurate: they are inappropriate. Can we imagine musical tones called lark, canary, cockatoo, crow, cat, dog, or mouse, because they bear some distant resemblance to the cries of those animals? See paragraph 131.

Color needs a system.

(3) Music is equipped with a system by which it defines each sound in terms of its pitch, intensify, and duration, without dragging in loose allusions to the endlessly varying sounds of nature. So should color be supplied with an appropriate system, based on the hue, value, and chroma2 of our sensations, and not attempting to describe them by the indefinite and varying colors of natural objects. The system now to be considered portrays the three dimensions of color, and measures each by an appropriate scale. It does not rest upon the whim of an individual, but upon physical measurements made possible by special color 11 apparatus. The results may be tested by any one who comes to the problem with “a clear mind, a good eye, and a fair supply of patience.”

Clear mental images make clear speech. Vague thoughts find vague utterance.

(4) The child gathers flowers, hoards colored beads, chases butterflies, and begs for the gaudiest painted toys. At first his strong color sensations are sufficiently described by the simple terms of red, yellow, green, blue, and purple. But he soon sees that some are light, while others are dark, and later comes to perceive that each hue has many grayer degrees. Now, if he wants to describe a particular red,—such as that of his faded cap,—he is not content to merely call it red, since he is aware of other red objects which are very unlike it. So he gropes for means to define this particular red; and, having no standard of comparison,—no scale by which to estimate,—he hesitatingly says it is a “sort of dull red.”

(5) Thus early is he cramped by the poverty of color language. He has never been given an appropriate word for this color quality, and has to borrow one signifying the opposite of sharp, which belongs to edge tools rather than to colors.

Most color terms are borrowed from other senses.

(6) When his older sister refers to the “tone” of her green dress, or speaks of the “key of color” in a picture, he is naturally confused, because tone and key are terms associated in his mind with music. It may not be long before he will hear that “a color note has been pitched too high,” or that a certain artist “paints in a minor key.” All these terms lead to mixed and indefinite ideas, and leave him unequipped for the clear expression of color qualities.

(7) Musical art is not so handicapped. It has an established 12 scale with measured intervals and definite terms. Likewise, coloristic art must establish a scale, measure its intervals, and name its qualities in unmistakable fashion.

Color has three dimensions.

(8) It may sound strange to say that color has three dimensions, but it is easily proved by the fact that each of them can be measured. Thus in the case of the boy’s faded cap its redness or HUE3 is determined by one instrument; the amount of light in the red, which is its VALUE,3 is found by another instrument; while still a third instrument determines the purity or CHROMA3 of the red.

The omission of any one of these three qualities leaves us in doubt as to the character of a color, just as truly as the character of this studio would remain undefined if the length were omitted and we described it as 22 feet wide by 14 feet high. The imagination would be free to ascribe any length it chose, from 25 to 100 feet. This length might be differently conceived by every individual who tried to supply the missing factor.

(9) To illustrate the tri-dimensional nature of colors. Suppose we peel an orange and divide it in five parts, leaving the sections slightly connected below (Fig. 4). Then let us say that all the reds we have ever seen are gathered in one of the sections, all yellows in another, all greens in the third, blues in the fourth, and purples in the fifth. Next we will assort these HUES in each section so that the lightest are near the top, and grade regularly to the darkest near the bottom. A white wafer connects all the sections at the top, and a black wafer may be added beneath. See Plate I.

13

(10) The fruit is then filled with assorted colors, graded from white to black, according to their VALUES, and disposed by their HUES in the five sections. A slice near the top will uncover light values in all hues, and a slice near the bottom will find dark values in the same hues. A slice across the middle discloses a circuit of hues all of MIDDLE VALUE; that is, midway between the extremes of white and black.

(11) Two color dimensions are thus shown in the orange, and it remains to exhibit the third, which is called CHROMA, or strength of color. To do this, we have only to take each section in turn, and, without disturbing the values already assorted, shove the grayest in toward the narrow edge, and grade outward to the purest on the surface. Each slice across the fruit still shows the circuit of hues in one uniform value; but the strong chromas are at the outside, while grayer and grayer chromas make a gradation inward to neutral gray at the centre, where all trace of color disappears. The thin edges of all sections unite in a scale of gray from black to white, no matter what hue each contains.

The curved outside of each section shows its particular hue graded from black to white; and, should the section be cut at right angles to the thin edge, it would show the third dimension,—chroma,—for the color is graded evenly from the surface to neutral gray. A pin stuck in at any point traces the third dimension.

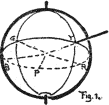
A color sphere can be used to unite the three dimensions of hue, value, and chroma.

(12) Having used the familiar structure of the orange as a help in classifying colors, let us substitute a geometric solid, like a sphere,4 and make use of geographical terms. The north pole is white. The south pole is black. 14 The equator is a circuit of middle reds, yellows, greens, blues, and purples. Parallels above the equator describe this circuit in lighter values, and parallels below trace it in darker values. The vertical axis joining black and white is a neutral scale of gray values, while perpendiculars to it (like a pin thrust into the orange) are scales of chroma. Thus our color notions may be brought into an orderly relation by the color sphere. Any color describes its light and strength by its location in the solid or on the surface, and is named by its place in the combined scales of hue, value, and chroma.

Two dimensions fail to describe a color.

(13) Much of the popular misunderstanding of color is caused by ignorance of these three dimensions or by an attempt to make two dimensions do the work of three.

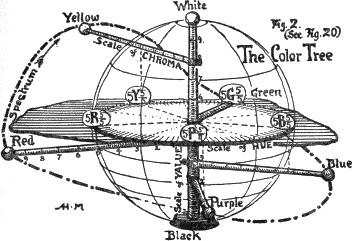
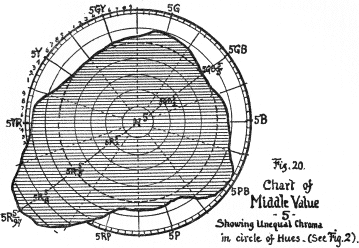
(14) Flat diagrams showing hues and values, but omitting to define chromas, are as incomplete as would be a map of Switzerland with the mountains left out, or a harbor chart without indications of the depth of water. We find by aid of the measuring instruments that pigments are very unequal in this third dimension,—chroma,—producing mountains and valleys on the color sphere, so that, when the color system is worked out in pigments and charted, some colors must be traced well out beyond the spherical surface (paragraphs 125–127). Indeed, a COLOR TREE5 is needed to display by the unequal levels and lengths of its branches the individuality of pigment colors. But, whatever solid or figure is used to illustrate color relations, it must combine the three scales of hue, value, and chroma, and these definite scales furnish a name for every color based upon its intrinsic qualities, and free from terms purloined in other sensations, or caught from the fluctuating colors of natural objects.

15

How this system describes the spectrum.

(15) The solar spectrum and rainbow are the most stimulating color experiences with which we are acquainted. Can they be described by this solid system?

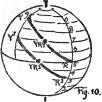
(16) The lightest part of the spectrum is a narrow field of greenish yellow, grading into darker red on one side and into darker green upon the other, followed by still darker blue and purple. Upon the sphere the values of these spectral colors trace a path high up on the yellow section, near white, and slanting downward across the red and green sections, which are traversed near the level of the equator, it goes on to cross the blue and purple well down toward black.

(17) This forms an inclined circuit, crossing the equator at opposite points, and suggests the ecliptic or the rings of Saturn (see outside cover). A pale rainbow would describe a slanting circuit nearer white, and a dimmer one would fall within the sphere, while an intensely brilliant spectrum projects far beyond the surface of the sphere, so greatly is the chroma of its hues in excess of the common pigments with which we work out our problems.

(18) At the outset it is well to recognize the place of the spectrum in this system, not only because it is the established basis of scientific study, but especially because the invariable order assumed by its hues is the only stable hint which Nature affords us in her infinite color play.

(19) All our color sensations are included in the color solid. None are left out by its scales of hue, value, and chroma. Indeed, the imagination is led to conceive and locate still purer colors than any we now possess. Such increased degrees of color sensation can be named, and clearly conveyed by symbols to another person as soon as the system is comprehended.

16

Appendix to Chapter I.

Misnomers for Color.

The Century Dictionary helps an intelligent study of color by its clear definitions and cross-references to HUE, VALUE, and CHROMA,—leaving no excuse for those who would confuse these three qualities or treat a degree of any quality as the quality itself.

Obscure statements were frequent in text-books before these new definitions appeared. Thus the term “shade” should be applied only to darkened values, and not to hues or chromas. Yet one writer says, “This yellow shades into green,” which is certainly a change of hue, and then speaks of “a brighter shade” in spite of his evident intention to suggest a stronger chroma, which is neither a shade nor brighter luminosity.

Children gain wrong notions of “tint and shade” from the so-called standard colors shown to them, which present “tints” of red and blue much darker than the “shades” of yellow. This is bewildering, and, like their elders, they soon drop into the loose habit of calling any degree of color-strength or color-light a “shade.” Value is a better term to describe the light which color reflects to the eye, and all color values, light or dark, are measured by the value-scale.

“Tone” is used in a confusing way to mean different things. Thus in the same sentence we see it refers to a single touch of the brush,—which is not a tone, but a paint spot,—and then we 17 read that the “tone of the canvas is golden.” This cannot mean that each paint spot is the color of gold, but is intended to suggest that the various objects depicted seem enveloped in a yellow atmosphere. Tone is, in fact, a musical term appropriate to sound, but out of place in color. It seems better to call the brush touch a color-spot: then the result of an harmonious relation between all the spots is color-envelope, or, as in Rood, “the chromatic composition.”

“Intensity” is a misleading term, if chroma be intended, for it depends on the relative light of spectral hues. It is a degree rather than a quality, as appears in the expressions, intense heat, light, sound,—intensity of stimulus and reaction. Being a degree of many qualities, it should not be used to describe the quality itself. The word becomes especially unfit when used to describe two very different phases of a color,—as its intense illumination, where the chroma is greatly weakened, and the strongest chroma which is found in a much lower value. “Purity” is also to be avoided in speaking of pigments, for not one of our pigments represents a single pure ray of the spectrum.

Examples are constantly found of the mental blur caused by such unfortunate terms, and, since misunderstanding becomes impossible with measured degrees of hue, value, and chroma, it seems only a question of time when they will take the place of tint, tone, shade, purity and intensity.

18

Chapter II.
COLOR QUALITIES.

(20) The three color qualities are hue, value, and chroma.

HUE is the name of a color.

(21) Hue is the quality by which we distinguish one color from another, as a red from a yellow, a green, a blue, or a purple. This names the hue, but does not tell whether it is light or dark, weak or strong,—leaving us in doubt as to its value and its chroma.

Science attributes this quality to difference in the LENGTH of ether waves impinging on the retina, which causes the sensation of color. The wave length M. 5269 gives a sensation of green, while M. 6867 gives a sensation of red.6

VALUE is the light of a color.

(22) Value is the quality by which we distinguish a light color from a dark one. Color values are loosely called tints and shades, but the terms are frequently misapplied. A tint should be a light value, and a shade should be darker; but the word “shade” has become a general term for any sort of color, so that a shade of yellow may prove to be lighter than a tint of blue. A photometric7 scale of value places all colors in relation to the extremes of white and black, but cannot describe their hue or their chroma.

19

Science describes this quality as due to difference in the HEIGHT or amplitude of ether waves impinging on the retina. Small amplitudes of the wave lengths given in paragraph 21 produce the sensation of dark green and dark red: larger amplitudes give the sensation of lighter green and lighter red.

CHROMA is the strength of a color.

(23) Chroma is the quality by which we distinguish a strong color from a weak one. To say that a rug is strong in color gives no hint of its hues or values, only its chromas. Loss of chroma is loosely called fading, but this word is frequently used to include changes of value and hue. Take two autumn leaves, identical in color, and expose one to the weather, while the other is waxed and pressed in a book. Soon the exposed leaf fades into a neutral gray, while the protected one preserves its strong chroma almost intact. If, in fading, the leaf does not change its hue or its value, there is only a loss of chroma, but the fading process is more likely to induce some change of the other two qualities. Fading, however, cannot define these changes.

Science describes chroma as the purity of one wave length separated from all others. Other wave lengths, INTERMINGLING, make its chroma less pure. A beam of daylight can combine all wave lengths in such balance as to give the sensation of whiteness, because no single wave is in excess.8

(24) The color sphere (see Fig. 1) is a convenient model to illustrate these three qualities,—hue, value, and chroma,—and unite them by measured scales.

(25) The north pole of the color sphere is white, and the south pole black. Value or luminosity of colors ranges between these two extremes. This is the vertical scale, to be memorized as V, 20 the initial for both value and vertical. Vertical movement through color may thus be thought of as a change of value, but not as a change of hue or of chroma. Hues of color are spread around the equator of the sphere. This is a horizontal scale, memorized as H, the initial for both hue and horizontal. Horizontal movement around the color solid is thus thought of as a change of hue, but not of value or of chroma. A line inward from the strong surface hues to the neutral gray axis, traces the graying of each color, which is loss of chroma, and conversely a line beginning with neutral gray at the vertical axis, and becoming more and more colored until it passes outside the sphere, is a scale of chroma, which is memorized as C, the initial both for chroma and centre. Thus the sphere lends its three dimensions to color description, and a color applied anywhere within, without, or on its surface is located and named by its degree of hue, of value, and of chroma.

HUES first appeal to the child, VALUES next, and CHROMAS last.

(26) Color education begins with ability to recognize and name certain hues, such as red, yellow, green, blue, and purple (see paragraphs 182 and 183). Nature presents these hues in union with such varieties of value and chroma that, unless there be some standard of comparison, it is impossible for one person to describe them intelligently to another.

(27) The solar spectrum forms a basis for scientific color analysis, taught in technical schools; but it is quite beyond the comprehension of a child. He needs something more tangible and constantly in view to train his color notions. He needs to handle colors, place them side by side for comparison, imitate them with 21 crayons, paints, and colored stuffs, so as to test the growth of perception, and learn by simple yet accurate terms to describe each by its hue, its value, and its chroma.

(28) Pigments, rather than the solar spectrum, are the practical agents of color work. Certain of them, selected and measured by this system (see Chapter V.), will be known as MIDDLE COLORS, because they stand midway in the scales of value and chroma. These middle colors are preserved in imperishable enamels,9 so that the child may handle and fix them in his memory, and thus gain a permanent basis for comparing all degrees of color. He learns to grade each middle color to its extremes of value and chroma.

(29) Experiments with crayons and paints, and efforts to match middle colors, train his color sense to finer perceptions. Having learned to name colors, he compares them with the enamels of middle value, and can describe how light or dark they are. Later he perceives their differences of strength, and, comparing them with the enamels of middle chroma, can describe how weak or strong they are. Thus the full significance of these middle colors as a practical basis for all color estimates becomes apparent; and, when at a more advanced stage he studies the best examples of decorative color, he will again encounter them in the most beautiful products of Oriental art.

22

Is it possible to define the endless varieties of color?

(30) At first glance it would seem almost hopeless to attempt the naming of every kind and degree of color. But, if all these varieties possess the same three qualities, only in different degrees, and if each quality can be measured by a scale, then there is a clue to this labyrinth.

A COLOR SPHERE and COLOR TREE to unite hue, value, and chroma.

(31) This clue is found in the union of these three qualities by measured scales in a color sphere and color tree.10 The equator of the sphere11 may be divided into ten parts, and serve as the scale of hue, marked R, YR, Y, GY, G, BG, B, PB, P, and RP. Its vertical axis may be divided into ten parts to serve as the scale of value, numbered from black (0) to white (10). Any perpendicular to the neutral axis is a scale of chroma. On the plane of the equator this scale is numbered 1, 2, 3, 4, 5, from the centre to the surface.

(32) This chroma scale may be raised or lowered to any level of value, always remaining perpendicular to the axis, and serving to measure the chroma of every hue at every level of value. The fact that some colors exceed others to such an extent as to carry them out beyond the sphere is proved by measuring instruments, 23 but the fact is a new one to many persons. (Figs. 2 and 3.)

(33) For this reason the COLOR TREE is a completer model than the sphere, although the simplicity of the latter makes it best for a child’s comprehension.

(34) The color tree is made by taking the vertical axis of the sphere, which carries a scale of value, for the trunk. The branches are at right angles to the trunk; and, as in the sphere, they carry the scale of chroma. Colored balls on the branches tell their Hue. In order to show the MAXIMA of color, each branch is attached to the trunk (or neutral axis) at a level demanded by its value,—the yellow nearest white at the top, then the green, red, blue, and purple branches, approaching black in the order of their lower values. It will be remembered that the chroma of the sphere ceased with 5 at the equator. The color tree prolongs 24 this through 6, 7, 8, and 9. The branch ends carry colored balls, representing the most powerful red, yellow, green, blue, and purple pigments which we now possess, and could be lengthened, should stronger chromas be discovered.12

(35) Such models set up a permanent image of color relations. Every point is self-described by its place in the united scales of hue, value, and chroma. These scales fix each new perception of color in the child’s mind by its situation in the color solid. The importance of such a definite image can hardly be overestimated, for without it one color sensation tends to efface another. When the child looks at a color, and has no basis of comparison, it soon leaves a vague memory that cannot be described. These models, on the contrary, lead to an intelligent estimate of each color in terms of its hue, its value, and its chroma; while the permanent enamels correct any personal bias by a definite standard.

(36) Thus defined, a color falls into logical relation with all other colors in the system, and is easily memorized, so that its image may be recalled at any distance of time or place by the notation.

(37) These solid models help to memorize and assemble colors and the memory is further strengthened by a simple NOTATION, which records each color so that it cannot be mistaken for any other. By these written scales a child gains an instinctive estimate of relations, so that, when he is delighted with a new color combination, its proportions are noted and understood.

(38) Musical art has long enjoyed the advantages of a definite scale and notation. Should not the art of coloring gain by similar definition? The musical scale is not left to personal 25 whim, nor does it change from day to day; and something as clear and stable would be an advantage in training the color sense.

(39) Perception of color is crude at first. The child sees only the most obvious distinctions, and prefers the strongest stimulation. But perception soon becomes refined by exercise, and, when a child tries to imitate the subtle colors of nature with paints, he begins to realize that the strongest colors are not the most beautiful,—rather the tempered ones, which may be compared to the moderate sounds in music. To describe these tempered colors, he must estimate their hue, value, and chroma, and be able to describe in what degree his copy departs from the natural color. And, with this gain in perception and imitation of natural color, he finds a strong desire to invent combinations to please his fancy. Thus the study divides into three related attitudes, which may be called recognition, imitation, and invention. Recognition of color is fundamental, but it would be tedious to spend a year or two in formal and dry exercises to train recognition of color alone; for each step in recognition of color is best tested by exercise in its imitation and arrangement. When perception becomes keener, emphasis can be placed on imitation of the colors found in art and in nature, resting finally on the selection and grouping of colors for design.13

Every color can be recognized, named, matched, imitated, and written by its HUE, VALUE, and CHROMA.

(40) The notation used in this system places Hue (expressed by an initial) at the left; Value (expressed by a number) at the right and above a line; and Chroma (also expressed by 26 a number) at the right, below the line. Thus R⁵/₉ means

HUE (red),	VALUE (5)	,	and will be found to represent the qualities of the pigment vermilion.14
	CHROMA (9)

Hue, value, and chroma unite in every color sensation, but the child cannot grasp them all at once. Hue-difference appeals to him first, and he gains a permanent idea of five principal hues from the enamels of MIDDLE COLORS, learning to name, match, imitate, and finally write them by their initials: R (red), Y (yellow), G (green), B (blue), and P (purple). Intermediates formed by uniting successive pairs are also written by the joined initials, YR (yellow-red), GY (green-yellow), BG (blue-green), PB (purple-blue), and RP (red-purple).

(41) Ten differences of hue are as many as a child can render at the outset, yet in matching and imitating them he becomes aware of their light and dark quality, and learns to separate it from hue as value-difference. Middle colors, as implied by that name, stand midway between white and black,—that is, on the equator of the sphere,—so that a middle red will be written R⁵/, suggesting the steps 6, 7, 8, and 9 which are above the equator, while steps 4, 3, 2, and 1 are below. It is well to show only three values of a color at first; for instance, the middle value contrasted with a light and a dark one. These are written R³/, R⁵/, R⁷/. Soon he perceives and can imitate finer differences, and the red scale may be written entire, as R¹/, R²/, R³/, R⁴/, R⁵/, R⁶/, R⁷/, R⁸/, R⁹/, with black as 0 and white as 10.

(42) Chroma-difference is the third and most subtle color quality. The child is already unconsciously familiar with the middle chroma of red, having had the enamels of MIDDLE COLOR always 27 in view, and the red enamel is to be contrasted with the strongest and weakest red chromas obtainable. These he writes R /₁, R /5, R /₉, seeing that this describes the chromas of red, but leaves out its values. R⁵/₁, R⁵/₅, R⁵/₉, is the complete statement, showing that, while both hue and value are unchanged, the chroma passes from grayish red to middle red (enamel first learned) and out to the strongest red in the chroma scale obtained by vermilion.

(43) It may be long before he can imitate the intervening steps of chroma, many children finding it difficult to express more than five steps of the chroma scale, although easily making ten steps of value and from twenty to thirty-five steps of hue. This interesting feature is of psychologic value, and has been followed in the color tree and color sphere.

Does such a scientific scheme leave any outlet for feeling and personal expression of beauty?

(44) Lest this exact attitude in color study should seem inartistic, compared with the free and almost chaotic methods in use, let it be said that the stage thus far outlined is frankly disciplinary. It is somewhat dry and unattractive, just as the early musical training is fatiguing without inventive exercises. The child should be encouraged at each step to exercise his fancy.

(45) Instead of cramping his outlook upon nature, it widens his grasp of color, and stores the memory with finer differences, supplying more material by which to express his sense of coloristic beauty.

(46) Color harmony, as now treated, is a purely personal affair, difficult to refer to any clear principles or definite laws. The very terms by which it seeks expression are borrowed from music, and suggest vague analogies that fail when put to the test. Color 28 needs a new set of expressive terms, appropriate to its qualities, before we can make an analysis as to the harmony or discord of our color sensations.

(47) This need is supplied in the present system by measured CHARTS, and a NOTATION. Their very construction preserves the balance of colors, as will be shown in the next chapter, while the chapter on harmony (Chapter VII.) shows how harmonious pairs and triads of color may be found by MASKS with measured intervals. In fact, practice in the use of the charts supplies the imagination with scales and sequences of color quite as definite and quite as easily written as those sound intervals by which the musician conveys to others his sense of harmony. And, although in neither art can training alone make the artist, yet a technical grasp of these formal scales gives acquaintance with the full range of the instrument, and is indispensable to artistic expression. From these color scales each individual is free to choose combinations in accord with his feeling for color harmony.

Let us make an outline of the course of color study traced in the preceding pages.15

PERCEPTION of color.

(48) Hue-difference.

Value-difference.

29

Chroma-difference.

EXPRESSION of color.

(49) Matching and imitation of hues (using stuffs, crayons, and paints).

Matching and imitation of values and hues (using stuffs, crayons, and paints).

Matching and imitation of chromas, values, and hues (using stuffs, crayons, and paints).

Notation of color.

Hue	Value	,  H	V	,
	Chroma		C

Initial for hue, numeral above for value, numeral below for chroma.

Sequences of color.

Balance of color.

30

HARMONY of color.

(50) Selection of colors that give pleasure.

Grouping of colors to suit some practical use: wall papers, rugs, book covers, etc.

A definite plan of color study, with freedom as to details of presentation.16

(51) Having memorized these broad divisions of the study, a clever teacher will introduce many a detail, to meet the mood of the class, or correlate this subject with other studies, without for a moment losing the thread of thought or befogging the presentation. But to range at random in the immense field of color sensations, without plan or definite aim in view, only courts fatigue of the retina and a chaotic state of mind.

(52) The same broad principles which govern the presentation of other ideas apply with equal force in this study. A little, well apprehended, is better than a mass of undigested facts. If the child is led to discover, or at least to think he is discovering, new things about color, the mind will be kept alert and seek out novel illustrations at every step. Now and then a pupil will be found 31 who leads both teacher and class by intuitive appreciation of color, and it is a subtle question how far such a nature can be helped or hurt by formal exercises. But such an exception is rare, and goes to prove that systematic discipline of the color sense is necessary for most children.

(53) Outdoor nature and indoor surroundings offer endless color illustrations. Birds, flowers, minerals, and the objects in daily use take on a new interest when their varied colors are brought into a conscious relation, and clearly named. A tri-dimensional perception, like this sense of color, requires skilful training, and each lesson must be simplified to the last point practicable. It must not be too long, and should lead to some definite result which a child can grasp and express with tolerable accuracy, while its difficulties should be approached by easy stages, so as to avoid failure or discouragement. The success of the present effort is the best incentive to further achievement.

32

Appendix to Chapter II.
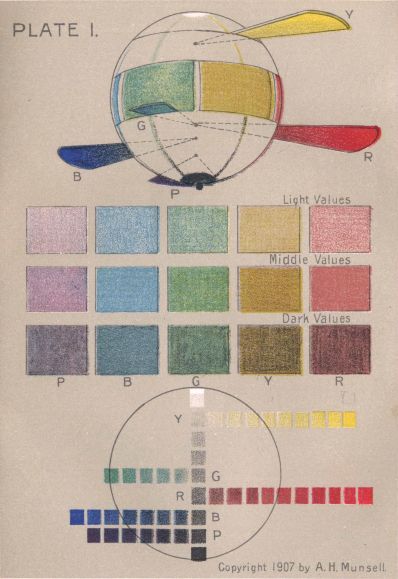
PLATE I.
THE COLOR SPHERE, with Measured Scales of
HUE, VALUE, and CHROMA.

The teacher of elementary grades introduces these scales of tempered color as fast as the child’s interest is awakened to their need by the exercises shown in Plates II. and III. Thus the Hue scale is learned before the end of the second year, the Value scale during the next two years, and the Chroma scale in the fifth year. By the time a child is ten years old these definite color scales have become part of his mental furnishing, so that he can name, write, and memorize any color group.

1. The Color Sphere in Skeleton. This diagram shows the middle colors on the equator, with strong red, yellow, green, blue, and purple, each at its proper level in the value scale, and projecting in accordance with its scale of chroma. See the complete description of these scales in Chapter II.

2. The Color Score. Fifteen typical steps taken from the color sphere are here spread out in a flat field. The Five Middle Colors form the centre level, with the same hues in a lighter value above and in a darker value below. Chapter VI. describes the making of this Score, and its use in analyzing colors and preserving a written record of their groups.

3. The Value Scale and Chroma Scale. Each of the five color maxima is thus shown at its proper level in the scale of light, and graded by uniform steps from its strongest chroma inward to neutrality at the axis of the sphere. Pigment inequalities here become very apparent.

---

33

FOR PLATES II. & III.,
SEE APPENDIX TO CHAPTER IV.,
CHILDREN’S COLOR STUDIES.

---

34

Chapter III.
COLOR MIXTURE AND BALANCE.

All colors grasped in the hand.

(54) 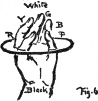Let us recall the names and order of colors given in the last chapter, with their assemblage in a sphere by the three qualities of HUE, VALUE, and CHROMA. It will aid the memory to call the thumb of the left hand RED, the forefinger YELLOW, the middle finger GREEN, the ring finger BLUE, and the little finger PURPLE (Fig. 6). When the finger tips are in a circle, they represent a circuit of hues, which has neither beginning nor end, for we can start with any finger and trace a sequence forward or backward. Now close the tips together for white, and imagine that the five strong hues have slipped down to the knuckles, where they stand for the equator of the color Sphere. Still lower down at the wrist is black.

(55) The hand thus becomes a color holder, with white at the finger tips, black at the wrist, strong colors around the outside, and weaker colors within the hollow. Each finger is a scale of its own color, with white above and black below, while the graying of all the hues is traced by imaginary lines which meet in the middle of the hand. Thus a child’s hand may be his substitute for the color sphere, and also make him realize that it is filled with grayer degrees of the outside colors, all of which melt into gray in the centre.

35

Neighborly and opposite hues; and their mixture.

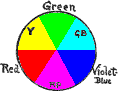
(56) 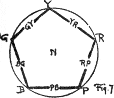Let this circle (Fig. 7) stand for the equator of the color sphere with the five principal hues (written by their initials R, Y, G, B, and P) spaced evenly about it. Some colors are neighbors, as red and yellow, while others are opposites. As soon as a child experiments with paints, he will notice the different results obtained by mixing them.

First, the neighbors, that is, any pair which lie next one another, as red and yellow, will unite to make a hue which retains a suggestion of both. It is intermediate between red and yellow, and we call it YELLOW-RED.17

(57) Green and yellow unite to form GREEN-YELLOW, blue and green make BLUE-GREEN, and so on with each succeeding pair. These intermediates are to be written by their initials, and inserted in their proper place between the principal hues. It is as if an orange (paragraph 9) were split into ten sectors instead of five, with red, yellow, green, blue, and purple as alternate sectors, while half of each adjoining color pair were united to form the sector between them. The original order of five hues is in no wise disturbed, but linked together by five intermediate steps.

(58) Here is a table of the intermediates made by mixing each pair:—

36

Using the left hand again to hold colors, the principal hues remain unchanged on the knuckles, but in the hollows between them are placed intermediate hues, so that the circle now reads: red, yellow-red, yellow, green-yellow, green, blue-green, blue, purple-blue, purple, and red-purple, back to the red with which we started. This circuit is easily memorized, so that the child may begin with any color point, and repeat the series clock wise (that is, from left to right) or in reverse order.

(59) Each principal hue has thus made two close neighbors by mixing with the nearest principal hue on either hand. The neighbors of red are a yellow-red on one side and a purple-red on the other. The neighbors of green are a green-yellow on one hand and a blue-green on the other. It is evident that a still closer neighbor could be made by again mixing each consecutive pair in this circle of ten hues; and, if the process were continued long enough, the color steps would become so fine that the eye could see only a circuit of hues melting imperceptibly one into another.

(60) But it is better for the child to gain a fixed idea of red, yellow, green, blue, and purple, with their intermediates, before attempting to mix pigments, and these ten steps are sufficient for primary education.

(61) Next comes the question of opposites in this circle. A line drawn from red, through the centre, finds its opposite, blue-green.18 If these colors are mixed, they unite to form gray. Indeed, the centre of the circle stands for a middle gray, not only because it is the centre of the neutral axis between black and white, but also because any pair of opposites will unite to form gray.

37

(62) This is a table of five mixtures which make neutral gray:

Opposites

Red & Yellow Green Blue Purple

Blue-green Purple-blue Red-purple Yellow-red Green-yellow

Each pair of which unites in neutral gray.

(63) But if, instead of mixing these opposite hues, we place them side by side, the eye is so stimulated by their difference that each seems to gain in strength; i.e., each enhances the other when separate, but destroys the other when mixed. This is a very interesting point to be more fully illustrated by the help of a color wheel in Chapter V., paragraph 106. What we need to remember is that the mixture of neighborly hues makes them less stimulating to the eye, because they resemble each other, while a mixture of opposite hues extinguishes both in a neutral gray.

Hues once removed, and their mixture.

(64) 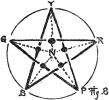There remains the question, What will happen if we mix, not two neighbors, nor two opposites, but a pair of hues once removed in the circle, such as red and green? A line joining this pair does not pass through the neutral centre, but to one side nearer yellow, which shows that this mixture falls between neutral gray and yellow, partaking somewhat of each. In the same way a line joining yellow and blue shows that their mixture contains both green and gray. Indeed, a line joining any two colors in the circuit may be said to describe their union. A radius crossing this line passes to some hue on the circumference, and describes by its intersection with the first line 38 the chroma of the color made by a mixture of the two original colors.

Red   & Yellow Green Blue Purple

Green make Blue Purple Red Yellow

Yellow‑gray Green-gray Blue-gray Purple‑gray Red-gray

Each pair unites in a colored gray, which is an intermediate hue of weak chroma.

Mixture of white and black: a scale of grays.

(65) So far we have thought only of the plane of the equator, with its circle of middle hues in ten steps, and studied their mixture by drawing lines to join them. Now let us start at the neutral centre, and think upward to white and downward to black (Fig. 9.)

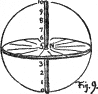
This vertical line is the neutral axis joining the poles of white and black, which represent the opposites of light and darkness. Middle gray is half-way between. If black is called 0, and white is 10, then the middle point is 5, with 6, 7, 8, and 9 above, while 4, 3, 2, and 1 are below, thus making a vertical scale of grays from black to white (Chapter II., paragraph 25).

If left to personal preference, an estimate of middle value will vary with each individual who attempts to make it. This appears in the neutral scales already published for schools, and students who depend upon them, discover a variation of over 10 per cent. in the selection of middle gray. Since this VALUE SCALE underlies all color work, it needs accurate adjustment by scientific means, as in scales of sound, of length, of weight, or of temperature.

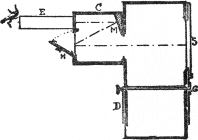
A PHOTOMETER (photo, light, and meter, a measure)19 is shown 39 on the next page. It measures the relative amount of light which the eye receives from any source, and so enables us to make a scale with any number of regular steps. The principle on which it acts is very simple.

A rectangular box, divided by a central partition into halves, has symmetrical openings in the front walls, which permit the light to reach two white fields placed upon the back walls. If one looks in through the observation tube, both halves are seen to be exactly alike, and the white fields equally illuminated. A valve is then fitted to one of the front openings, so that the light in that half of the photometer may be gradually diminished. Its white field is thus darkened by measured degrees, and becomes black when all light is excluded by the closed valve. While this darkening process goes on in one-half of the instrument, the white field in the other half does not change, and, looking into the eyepiece, the observer sees each step contrasted with the original white. One-half is thus said to be variable because of its valve, and the other side is said to be fixed. A dial connected with the valve has a hand moving over it to show how much light is admitted to the field in the variable half.

Let us now test one of these personal decisions about middle value. A sample replaces the white field in the fixed half, and by means of the valve, the white field in the variable half is alternately darkened and lightened, until it matches the sample and the eye sees no difference in the two. The dial then discloses the fact that this supposedly MIDDLE VALUE reflects only 42 per cent. of the light; that is to say, it is nearly a whole step too low in a decimal scale. Other samples err nearly as far on the light side of middle value, and further tests prove not only the varying color sensitiveness of individuals, but detect a difference between the left and right eye of the same person.

40

PHOTOMETER.

Back View.	Front View.

The vagaries of color estimate thus disclosed, lead some to seek shelter in “feeling and inspiration”; but feeling and inspiration are temperamental, and have nothing to do with the simple facts of vision. A measured and unchanging scale is as necessary and valuable in the training of the eye as the musical scale in the discipline of the ear.

It will soon be necessary to talk of the values in each color. We may distinguish the values on the neutral axis from color values by writing them N¹, N², N³, N⁴, N⁵, N⁶, N⁷, N⁸, N⁹, N¹⁰. Such a scale makes it easy to foresee the result of mixing light values with dark ones. Any two gray values unite to form a gray midway between them. Thus N⁴ and N⁶ being equally above and below the centre, unite to form N⁵, as will also N⁷ 41 and N³, N⁸ and N², or N⁹ and N¹. But N⁹ and N³ will unite to form N⁶, which is midway between 6 and 9.

Vertical Section through light openings.

PARTS.

(66) When this numbered scale of values is familiar, it serves not only to describe light and dark grays, but the value of colors which are at the same level in the scale. Thus R⁷ (popularly called a tint of red) is neither lighter nor darker than the gray of N⁷. A numeral written above to the right always indicates value, whether of a gray or a color, so that R¹, R², R³, R⁴, R⁵, R⁶, R⁷, R⁸, R⁹, describes a regular scale of red values from black to white, while G¹, G², G³, etc., is a scale of green values.

42

(67) This matter of a notation for colors will be more fully worked out in Chapter VI., but the letters and numerals already described greatly simplify what we are about to consider in the mixture and balance of colors.

Mixture of light hues with dark hues.

(68) Now that we are supplied with a decimal scale of grays, represented by divisions of the neutral axis (N¹, N², etc.), and a corresponding decimal scale of value for each of the ten hues ranged about the equator (R¹, R²,—YR¹, YR²,—Y¹, Y²,—GY¹, GY²,—and so on), traced by ten equidistant meridians from black to white, it is not difficult to foresee what the mixture of any two colors will produce, whether they are of the same level of value, as in the colors of the equator already considered, or whether they are of different levels.

(69) For instance, let us mix a light yellow (Y⁷) with a dark red (R³). They are neighbors in hue, but well removed in value. A line joining them centres at YR⁵. This describes the result of their mixture,—a value intermediate between 7 and 3, with a hue intermediate between R and Y. It is a yellow-red of middle value, popularly called “dark orange.” But, while this term “dark orange” rarely means the same color to three different people, these measured scales give to YR⁵ an unmistakable meaning, just as the musical scale gives an unmistakable significance to the notes of its score.

(70) Evidently, this way of writing colors by their degrees of value and hue gives clearness to what would otherwise be hard to express by the color terms in common use.

(71) If Y⁹ and R⁵ be chosen for mixture, we know at once that 43 they unite in YR⁷, which is two steps of the value scale above the middle; while Y⁶ and R² make YR⁴, which is one step below the middle. Charts prepared with this system show each of these colors and their mixture with exactness.

(72) The foregoing mixtures of dark reds and light yellows are typical of the union of light and dark values of any neighboring hues, such as yellow and green, green and blue, blue and purple, or purple and red. Next let us think of the result of mixing different values in opposite hues; as, for instance, YR⁷ and B³ (Fig. 11). To this combination the color sphere gives a ready answer; for the middle of a straight line through the sphere, and joining them, coincides with the neutral centre, showing that they balance in neutral gray. This is also true of any opposite pair of surface hues where the values are equally removed from the equator.

(73) 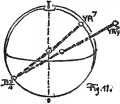Suppose we substitute familiar flowers for the notation, then YR⁷ becomes the buttercup, and B³ is the wild violet. But, in comparing the two, the eye is more stimulated by the buttercup than by the violet, not alone because it is lighter, but because it is stronger in chroma; that is, farther away from the neutral axis of the sphere, and in fact out beyond its surface, as shown in Fig. 11.

The head of a pin stuck in toward the axis on the 7th level of YR may represent the 9th step in the scale of chroma, such as the buttercup, while the “modest” violet with a chroma of only 4, is shown by its position to be nearer the neutral axis than the brilliant buttercup by five steps of chroma. This is the third dimension of color, and must be included in our notation. 44 So we write the buttercup YR⁷/₉ and the violet B³/₄,—chroma always being written below to the right of hue, and value always above.

(This is the invariable order: HUE	VALUE	.)
	CHROMA

(74) A line joining the head of the pin mentioned above with B³/₄ does not pass through the centre of the sphere, and its middle point is nearer the buttercup than the neutral axis, showing that the hues of the buttercup and violet do not balance in gray.

The neutral centre is a balancing point for colors.

(75) This raises the question, What is balance of color? Artists criticise the color schemes of paintings as being “too light or too dark” (unbalanced in value), “too weak or too strong” (unbalanced in chroma), and “too hot or too cold” (unbalanced in hue), showing that this is a fundamental idea underlying all color arrangements.

(76) Let us assume that the centre of the sphere is the natural balancing point for all colors (which will be best shown by Maxwell discs in Chapter V., paragraphs 106–112), then color points equally removed from the centre must balance one another. Thus white balances black. Lighter red balances darker blue-green. Middle red balances middle blue-green. In short, every straight line through this centre indicates opposite qualities that balance one another. The color points so found are said to be “complementary,” for each supplies what is needed to complement or balance the other in hue, value, and chroma.

(77) The true complement of the buttercup, then, is not the violet, which is too weak in chroma to balance its strong opposite. We have no blue flower that can equal the chroma of the buttercup. Some other means must be found to produce a balance. One way is to use more of the weaker color. Thus we can make 45 a bunch of buttercups and violets, using twice as many of the latter, so that the eye sees an area of blue twice as great as the area of yellow-red. Area as a compensation for inequalities of hue, value, and chroma will be further described under the harmony of color in Chapter VII.

(78) But, before leaving this illustration of the buttercup and violet, it is well to consider another color path connecting them which does not pass through the sphere, but around it (Fig. 12). Such a path swinging around from yellow-red to blue slants downward in value, and passes through yellow, green-yellow, green, and blue-green, tracing a sequence of hue, of which each step is less chromatic than its predecessor.

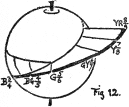
This diminishing sequence is easily written thus,—YR⁸/₉, Y⁷/₈, GY⁶/₇, G⁵/₆, BG⁴/₅, B³/₄,—and is shown graphically in Fig. 12. Its hue sequence is described by the initials YR, Y, GY, G, BG, and B. Its value-sequence appears in the upper numerals, 8, 7, 6, 5, 4, and 3, while the chroma-sequence is included in the lower numerals, 9, 8, 7, 6, 5, and 4. This gives a complete statement of the sequence, defining its peculiarity, that at each change of hue there is a regular decrease of value and chroma. Nature seems to be partial to this sequence, constantly reiterating it in yellow flowers with their darker green leaves and underlying shadows. In spring time she may contract its range, making the blue more green and the yellow less red, but in autumn she seems to widen the range, presenting strong contrasts of yellow-red and purple-blue.

(79) Every day she plays upon the values of this sequence, 46 from the strong contrasts of light and shadow at noon to the hardly perceptible differences at twilight. The chroma of this sequence expands during the summer to strong colors, and contracts in winter to grays. Indeed, Nature, who would seem to be the source of our notions of color harmony, rarely repeats herself, yet is endlessly balancing inequalities of hue, value, and chroma by compensations of quantity.

(80) So subtle is this equilibrium that it is taken for granted and forgotten, except when some violent disturbance disarranges it, such as an earthquake or a thunder-storm.

The triple nature of color balance illustrated.

(81) The simplest idea of balance is the equilibrium of two halves of a stick supported at its middle point. If one end is heavier than the other, the support must be moved nearer to that end.

But, since color unites three qualities, we must seek some type of triple balance. The game of jackstraws illustrates this, when the disturbance of one piece involves the displacement of two others. The action of three children on a floating plank or the equilibrium of two acrobats carried on the shoulders of a third may also serve as examples.

(82) Triple balance may be graphically shown by three discs in contact. Two of them are suspended by their centres, while they remain in touch with a third supported on a pivot, as in Fig. 14. Let us call the lowest disc Hue (H), and the lateral discs Value (V) and Chroma (C). Any dip or rotation of the lower disc H will induce sympathetic action in the two lateral discs V and C. When H is inclined, both V 47 and C change their relations to it. If H is raised vertically, both V and C dip outward. If H is rotated, both V and C rotate, but in opposite directions. Indeed, any disturbance of V affects H and C, while H and V respond to any movement of C. So we must be prepared to realize that any change of one color quality involves readjustment of the other two.

(83) Color balance soon leads to a study of optics in one direction, to æsthetics in another, and to mathematical proportions in a third, and any attempt at an easy solution of its problems is not likely to succeed. It is a very complicated question, whose closest counterpart is to be sought in musical rhythms. The fall of musical impulses upon the ear can make us gay or sad, and there are color groups which, acting through the eye, can convey pleasure or pain to the mind.

(84) A colorist is keenly alive to these feelings of satisfaction or annoyance, and consciously or unconsciously he rejects certain combinations of color and accepts others. Successful pictures and decorative schemes are due to some sort of balance uniting “light and shade” (value), “warmth and coolness” (hue), with “brilliancy and grayness” (chroma); for, when they fail to please, the mind at once begins to search for the unbalanced quality, and complains that the color is “too hot,” “too dark,” or “too crude.” This effort to establish pleasing proportions may be unconscious in one temperament, while it becomes a matter of definite analysis in another. Emerson claimed that the unconscious only is complete. We gladly permit those whose color instinct is unerring—(and how few they are!)—to neglect all rules and set formulas. But education is concerned with the many who have not this gift.

(85) Any real progress in color education must come not from a blind imitation of past successes, but by a study into the laws 48 which they exemplify. To exactly copy fine Japanese prints or Persian rugs or Renaissance tapestries, while it cultivates an appreciation of their refinements, does not give one the power to create things equally beautiful. The masterpieces of music correctly rendered do not of necessity make a composer. The musician, besides the study of masterpieces, absorbs the science of counterpoint, and records by an unmistakable notation the exact character of any new combination of musical intervals which he conceives.

(86) So must the art of the colorist be furnished with a scientific basis and a clear form of color notation. This will record the successes and failures of the past, and aid in a search, by contrast and analysis, for the fundamentals of color balance. Without a measured and systematic notation, attempts to describe color harmony only produce hazy generalities of little value in describing our sensations, and fail to express the essential differences between “good” and “bad” color.

49

Appendix to Chapter III.

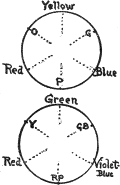
False Color Balance. There is a widely accepted error that red, yellow, and blue are “primary,” although Brewster’s theory was long ago dropped when the elements of color vision proved to be RED, GREEN, and VIOLET-BLUE. The late Professor Rood called attention to this in Chapters VIII.–XI. of his book, “Modern Chromatics,” which appeared in 1879. Yet we find it very generally taught in school. Nor does the harm end there, for placing red, yellow, and blue equidistant in a circle, with orange, green, and purple as intermediates, the teacher goes on to state that opposite hues are complementary.

Red is	thus made the complement of	Green,
Yellow	„ „	Purple, and
Blue	„ „	Orange.

Unfortunately, each of these statements is wrong, and, if tested by the mixture of colored lights or with Maxwell’s rotating discs, their falsity is evident.

There can be no doubt that green is not the complement of red, nor purple of yellow, nor orange of blue, for neither one of these pairs unites as it should in a balanced neutrality, and a total test of the circle gives great excess of orange, showing that red 50 and yellow usurp too great a portion of the circumference. Starting from a false basis, the Brewster theory can only lead to unbalanced and inharmonious effects of color.

The fundamental color sensations are RED, GREEN, and VIOLET-BLUE.

Red has for its	true complement	BLUE-GREEN,
Green	„„	RED-PURPLE, and
Violet-blue	„„	YELLOW,

all of the hues in the right-hand column being compound sensations. The sensation of green is not due to a mixture of yellow and blue, as the absorptive action of pigments might lead one to think: GREEN IS FUNDAMENTAL, and not made by mixing any hues of the spectrum, while YELLOW IS NOT FUNDAMENTAL, but caused by the mingled sensations of red and green. This is easily proved by a controlled spectrum, for all yellow-reds, yellows, and green-yellows can be matched by certain proportions of red and green light, all blue-greens, blues, and purple-blues can be obtained by the union of green and violet light, while purple-blue, purple, and red-purple result from the union of violet and red light. But there is no point where a mixture gives red, green, or violet-blue. They are the true primaries, whose mixtures produce all other hues.

Studio and school-room practice still cling to the discredited theory, claiming that, if it fails to describe our color sensations, yet it may be called practically true of pigments, because a red, yellow, and blue pigment suffice to imitate most natural colors. This discrepancy between pigment mixture and retinal mixture becomes clear as soon as one learns the physical make-up and behavior of paints.

51

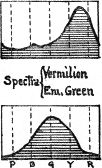Spectral analysis shows that no pigment is a pure example of the dominant hue which it sends to the eye. Take, for example, the very chromatic pigments representing red and green, such as vermilion and emerald green. If each emitted a single pure hue free from trace of any other hue, then their mixture would appear yellow, as when spectral red and green unite. But, instead of yellow, their mixture produces a warm gray, called brown or “dull salmon,” and this is to be inferred from their spectra, where it is seen that vermilion emits some green and purple as well as its dominant color, while the green also sends some blue and red light to the eye.20

Thus stray hues from other parts of the spectrum tend to neutralize the yellow sensation, which would be strong if each of the pigments were pure in the spectral sense. Pigment absorption affects all palette mixtures, and, failing to obtain a satisfactory yellow by mixture of red and green, painters use original yellow pigments,—such as aureolin, cadmium, and lead chromate,—each of them also impure but giving a dominant sensation of yellow. Did the eye discriminate, as does the ear when it analyzes the separate tones of a chord, then we should recognize that yellow pigments emit both red and green rays.

White light dispersed into a colored band by one prism, may have the process reversed by a second prism, so that the eye sees again only white light. But this would not be so, did not the balance of red, green, and violet-blue sensations remain undisturbed. All our ideas of color harmony are based upon this fundamental relation, and, if pigments are to render harmonious effects, 52 we must learn to control their impurities so as to preserve a balance of red, green, and violet-blue.

Otherwise, the excessive chroma and value of red and yellow pigments so overwhelm the lesser degrees of green and blue pigments that no balance is possible, and the colorist of fine perception must reject not alone the theoretical, but also the practical outcome of a “red-yellow-blue” theory.

Some of the points raised in this discussion are rather subtle for students, and may well be left until they arise in a study of optics, but the teacher should grasp them clearly, so as not to be led into false statements about primary and complementary hues.

53

Chapter IV.
PRISMATIC COLOR.

Pure color is seen in the spectrum of sunlight.

(87) The strongest sensation of color is gained in a darkened room, with a prism used to split a beam of sunlight into its various wave lengths. Through a narrow slit there enters a straight pencil of light which we are accustomed to think of as white, although it is a bundle of variously colored rays (or waves of ether) whose union and balance is so perfect that no single ray predominates.

(88) 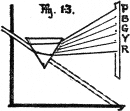Cover the narrow slit, and we are plunged in darkness. Admit the beam, and the eye feels a powerful contrast between the spot of light on the floor and its surrounding darkness. Place a triangular glass prism near the slit to intercept the beam of white light, and suddenly there appears on the opposite wall a band of brilliant colors. This delightful experiment rivets the eye by the beauty and purity of its hues. All other colors seem weak by comparison.

Their weakness is due to impurity, for all pigments and dyes reflect portions of hues other than their dominant one, which tend to “gray” and diminish their chroma.

(89) But prismatic color is pure, or very nearly so, because the shape of the glass refracts each hue, and separates it by the length 54 of its ether wave. These waves have been measured, and science can name each hue by its wave length. Thus a certain red is known as M. 6867, and a certain green sensation is M. 5269.21 Without attempting any scientific analysis of color, let it be said that Sir Isaac Newton made his series of experiments in 1687, and was privileged to name this color sequence by seven steps which he called red, orange, yellow, green, blue, violet, and indigo. Later a scientist named Fraunhofer discovered fine black lines crossing the solar spectrum, and marked them with letters of the alphabet from a to h. These with the wave length serve to locate every hue and define every step in the sequence. Since Newton’s time it has been proved that only three of the spectral hues are primary; viz., a red, a green, and a violet-blue, while their mixture produces all other gradations. By receiving the spectrum on an opaque screen with fine slits that fit the red and green waves, so that they alone pass through, these two primary hues can be received on mirrors inclined at such an angle as to unite on another screen, where, instead of red or green, the eye sees only yellow.22

(90) A similar arrangement of slits and mirrors for the green and violet-blue proves that they unite to make blue, while a third experiment shows that the red and violet-blue can unite to make purple. So yellow, blue-green, and purple are called secondary 55 hues because they result from the mixture of the three primaries, red, green, and violet-blue.

In comparing these two color lists, we see that the “indigo” and “orange” of Sir Isaac Newton have been discarded. Both are indefinite, and refer to variable products of the vegetable kingdom. Violet is also borrowed from the same kingdom; and, in order to describe a violet, we say it is a purple violet or blue violet, as the case may be, just as we describe an orange as a red orange or a yellow orange. Their color difference is not expressed by the terms “orange” or “violet,” but by the words “red,” “yellow,” “blue,” or “purple,” all of which are true color names and arouse an unmixed color image.

(91) In the nursery a child learns to use the simple color names red, yellow, green, blue, and purple. When familiarity with the color sphere makes him relate them to each other and place them between black and white by their degree of light and strength, there will be no occasion to revert to vegetables, animals, minerals, or the ever-varying hues of sea and sky to express his color sensations.

(92) Another experiment accentuates the difference between spectral and pigment color. When the spectrum is spread on the screen by the use of a prism, and a second prism is placed inverted beyond the first, it regathers the dispersed rays back into their original beam, making a white spot on the floor. This proves that all the colored rays of light combine to balance each other in whiteness. But if pigments which are the closest possible imitation of these hues are united on a painter’s palette, either by the brush or the knife, they make gray, and not white.

(93) This is another illustration of the behavior of pigments, for, instead of uniting to form white, they form gray, which is a 56 darkened or impure form of white; and, lest this should be attributed to a chemical reaction between the various matters that serve as pigments, the experiment can be carried out without allowing one pigment to touch another by using Maxwell discs, as will be shown in the next chapter.

(94) Before leaving these prismatic colors, let us study them in the light of what has already been learned of color dimensions. Not only do they present different values, but also different chromas. Their values range from darkness at each end, where red and purple become visible, to a brightness in the greenish yellow, which is almost white. So on the color tree described in Chapter II., paragraph 34, yellow has the highest branch, green is lower, red is below the middle, with blue and purple lower down, near black.

(95) Then in chroma they range from the powerful stimulation of the red to the soothing purple, with green occupying an intermediate step. This is also given on the color tree by the length of its branches.

(96) 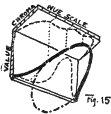In Fig. 15 the vertical curve describes the values of the spectrum as they grade from red through yellow, green, blue, and purple. The horizontal curve describes the chromas of the spectrum in the same sequence; while the third curve leaning outward is obtained by uniting the first two by two planes at right angles to one another, and sums up the three qualities by a single descriptive line. Now the red and purple ends are far apart, and science forbids their junction because of their great difference in wave length. But the mind is prone to unite them in order to produce the red-purples which we see in clouds at sunset, in flowers and 57 grapes and the amethyst. Indeed, it has been done unhesitatingly in most color schemes in order to supply the opposite of green.

(97) This gives a slanting circuit joining all the branch ends of the color tree, and has been likened to the rings of Saturn in Chapter I., paragraph 17.

A prismatic color sphere.

(98) With a little effort of the imagination we can picture a prismatic color sphere, using only the colors of light. In a cylindrical chamber is hung a diaphanous ball similar to a huge soap bubble, which can display color on its surface without obscuring its interior. Then, at the proper points of the surrounding wall, three pure beams of colored light are admitted,—one red, another green, and the third violet-blue.

(99) They fall at proper levels on three sides of the sphere, while their intermediate gradations encircle the sphere with a complete spectrum plus the needed purple. As they penetrate the sphere, they unite to balance each other in neutrality. Pure whiteness is at the top, and, by some imaginary means their light gradually diminishes until they disappear in darkness below.

(100) This ideal color system is impossible in the present state of our knowledge and implements. Even were it possible, its immaterial hues could not serve to dye materials or paint pictures. Pigments are, and will in all probability continue to be, the practical agents of coloristic productions, however reluctant the scientist may be to accept them as the basis of a color system. It is true that they are chemically impure and imperfectly represent the colors of light. Some of them fade rapidly and undergo chemical change, as in the notable case of a green pigment tested 58 by this measured system, which in a few weeks lost four steps of chroma, gained two steps of value, and swung into a bluer hue.

(101) But the color sphere to be next described is worked out with a few reliable pigments, mostly natural earths, whose fading is a matter of years and so slight as to be almost imperceptible. Besides, its principal hues are preserved in safe keeping by imperishable enamels, which can be used to correct any tendency of the pigments to distort the measured intervals of the color sphere.

This meets the most serious objection to a pigment system. Without it a child has nothing tangible which he can keep in constant view to imitate and memorize. With it he builds up a mental image of measured relations that describe every color in nature, including the fleeting hues of the rainbow, although they appear but for a moment at rare intervals. Finally, it furnishes a simple notation which records every color sensation by a letter and two numerals. With the enlargement of his mental power he will unite these in a comprehensive grasp of the larger relations of color.

59

Appendix to Chapter IV.

Children’s Color Studies.

These reproductions of children’s work are given as proof that color charm and good taste may be cultivated from the start.

Five Middle Hues are first taught by the use of special crayons, and later with water colors. They represent the equator of the color sphere (see Plate I.),—a circle midway between the extremes of color-light and color-strength,—and are known as Middle Red, Middle Yellow, Middle Green, Middle Blue, and Middle Purple.

These are starting-points for training the eye to measure regular scales of Value and Chroma.23 Only with such a trained judgment is it safe to undertake the use of strong colors.24

Beginners should avoid Strong Color. Extreme red, yellow, and blue are discordant. (They “shriek” and “swear.” Mark Twain calls Roxana’s gown “a volcanic eruption of infernal splendors.”) Yet there are some who claim that the child craves them, and must have them to produce a thrill. So also does he crave candies, matches, and the carving-knife. He covets the trumpet, fire-gong, and bass-drum for their “thrill”; but who would think them necessary 60 to the musical training of the ear? Like the blazing bill-board and the circus wagon, they may be suffered out-of-doors; but such boisterous sounds and color sprees are unfit for the school-room.

Quiet Color is the Mark of Good Taste. Refinement in dress and the furnishings of the home is attractive, but we shrink from those who are “loud” in their speech or their clothing. If we wish our children to become well-bred, is it logical to begin by encouraging barbarous tastes? Their young minds are very open to suggestion. They quickly adopt our standards, and the blame must fall upon us if they acquire crude color habits. Yellow journalism and rag-time tunes will not help their taste in speech or song, nor will violent hues improve their taste in matters of color.

Balance of Color is to be sought. Artists and decorators are well aware of a fact that slowly dawns upon the student; namely, that color harmony is due to the preservation of a subtle balance and impossible by the use of extremes. This balance of color resides more within the spherical surface of this system than in the excessive chromas which project beyond. It is futile to encourage children in efforts to rival the poppy or buttercup, even with the strongest pigments obtainable. Their sunlit points give pleasure because they are surrounded and balanced by blue ether and wide green fields. Were these conditions reversed, so that the flowers appeared as little spots of blue or green in great fields of blazing red, orange, and yellow, our pained eyes would be shut in disgust.

The painter knows that pigments cannot rival the brilliancy of the buttercup and poppy, enhanced by their surroundings. What is more, he does not care to attempt it. Nor does the musician wish to imitate the screech of a siren or the explosion of a gun. These are not subjects for art. Harmonious sounds are the study of the musician, and tuned colors are the materials of the colorist. Corot 61 in landscape, and Titian, Velasquez, and Whistler in figure painting, show us that Nature’s richest effects and most beautiful color are enveloped in an atmosphere of gray.

Beauty of Color lies in Tempered Relations. Music rarely touches the extreme range of sound, and harmonious color rarely uses the extremes of color-light or color-strength. Regular scales in the middle register are first given to train the ear, and so should the eye be first familiarized with medium degrees of color.

This system provides measured scales, established by special instruments, and is able to select the middle points of red, yellow, green, blue, and purple as a basis for comparing and relating all colors. These five middle colors form a Chromatic Tuning Fork. (See page 70.) It is far better that children should first become familiar with these tuned color intervals which are harmonious in themselves rather than begin by blundering among unrelated degrees of harsh and violent color. Who would think of teaching the musical scale with a piano out of tune?

The Tuning of Color cannot be left to Personal Whim. The wide discrepancies of red, yellow, and blue, which have been falsely taught as primary colors, can no more be tuned by a child than the musical novice can tune his instrument. Each of these hues has three variable factors (see page 14, paragraph 14), and scientific tests are necessary to measure and relate their uneven degrees of Hue, Value, and Chroma.

Visual estimates of color, without the help of any standard for comparison, are continually distorted by doubt, guess-work, and the fatigue of the eye. Hardly two persons can agree in the intelligible description of color. Not only do individuals differ, but the same eye will vary in its estimates from day to day. A frequent assumption that all strong pigments are equal in chroma, is 62 far from the truth, and involves beginners in many mishaps. Thus the strongest blue-green, chromium sesquioxide, is but half the chroma of its red complement, the sulphuret of mercury. Yet ignorance is constantly leading to their unbalanced use. Indeed, some are still unaware that they are the complements of each other.25

It is evident that the fundamental scales of Hue, Value, and Chroma must be established by scientific measures, not by personal bias. This system is unique in the possession of such scales, made possible by the devising of special instruments for the measurement of color, and can therefore be trusted as a permanent basis for training the color sense.

The examples in Plates II. and III. show how successfully the tuned crayons, cards, and water colors of this system lead a child to fine appreciations of color harmony.

PLATE II.
Color Studies with TUNED CRAYONS
in the Lower Grades.

Children have made every example on this plate, with no other material than the five crayons of middle hue, tempered with gray and black. A Color Sphere is always kept in the room for reference, and five color balls to match the five middle hues are placed in the hands of the youngest pupils. Starting with these middle points in the scales of Value and Chroma, they learn to estimate rightly all lighter and darker values, all weaker and stronger chromas, and gradually build up a disciplined judgment of color.

63

Each study can be made the basis of many variations by a simple change of one color element, as suggested in the text.

For principles involved in these color groups, see Chapter III.

PLATE III.
Color Studies with TUNED WATER COLORS
in the Upper Grades.

Previous work with measured scales, made by the tuned crayons and tested by reference to the color sphere, have so trained the color judgment that children may now be trusted with more flexible material. They have memorized the equable degrees of color on the equator of the sphere, and found how lighter colors may balance darker colors, how small areas of stronger chroma may be balanced by larger masses of weaker chroma, and in general gained a disciplined color sense. Definite impressions and clear thinking have taken the place of guess-work and blundering.

Thus, before reaching the secondary school, they are put in possession of the color faculty by a system and notation similar to that which was devised centuries ago for the musical sense. No system, however logical, will produce the artist, but every artist needs some systematic training at the outset, and this simple method by measured scales is believed to be the best yet devised.

Each example on this plate may be made the basis of many variants, by small changes in the color steps, as suggested in the 65 text, and further elaborated in Chapter VI. Indeed, the studies reproduced on Plates II. and III. are but a handful among hundreds of pleasing results produced in a single school.26

For analysis of the groups and means of recording them, see Chapter III.

66

Chapter V.
A PIGMENT COLOR SPHERE.27

How to make a color sphere with pigments.

(102) The preceding chapters have built up an ideal color solid, in which every sensation of color finds its place and is clearly named by its degree of hue, value, and chroma.

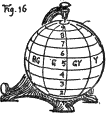
It has been shown that the neutral centre of the system is a balancing point for all colors, that a line through this centre finds opposite colors which balance and complement each other; and we are now ready to make a practical application, carrying out these ideal relations of color as far as pigments will permit in a color sphere27 (Fig. 16).

(103) The materials are quite simple. First a colorless globe, mounted so as to spin freely on its axis. Then a measured scale of value, specially devised for this purpose, obtained by the daylight photometer.28 Next a set of carefully chosen pigments, whose reasonable permanence has been tested by long use, and which are prepared so that they will not glisten when spread on the surface of the globe, but give a uniformly mat surface. A glass palette, palette knife, and some fine brushes complete the list.

(104) Here is a list of the paints arranged in pairs to represent 67 the five sets of opposite hues described in Chapter III., paragraphs 61–63:—

Color Pairs.	Pigments Used.	Chemical Nature.
Red    and	Venetian red.	Calcined native earth.
Blue-green.	Viridian and Cobalt.	Chromium sesquioxide.
Yellow    and	Raw Sienna.	Native earth.
Purple-blue.	Ultramarine.	Artificial product.
Green    and	Emerald green.	Arsenate of copper.
Red-purple.	Purple madder.	Extract of the madder plant.
Blue    and	Cobalt.	Oxide of cobalt with alumina.
Yellow-red.	Orange cadmium.	Sulphide of cadmium.
Purple    and	Madder and cobalt.	See each pigment above.
Green-yellow.	Emerald green and Sienna.	See each pigment above.

(105) These paints have various degrees of hue, value, and chroma, but can be tempered by additions of the neutrals, zinc white and ivory black, until each is brought to a middle value and tested on the value scale. After each pair has been thus balanced, they are painted in their appropriate spaces on the globe, forming an equator of balanced hues.

(106) The method of proving this balance has already been suggested in Chapter IV., paragraph 93. It consists of an ingenious implement devised by Clerk-Maxwell, which gives us a result of mixing colors without the chemical risks of letting them come in contact, and also measures accurately the quantity of each which is used (Fig. 17).

(107) This is called a Maxwell disc, and is nothing more than 68 a circle of firm cardboard, pierced with a central hole to fit the spindle of a rotary motor, and with a radial slit from rim to centre, so that another disc may be slid over the first to cover any desired fraction of its surface. Let us paint one of these discs with Venetian red and the other with viridian and cobalt, the first pair in the list of pigments to be used on the globe.

(108) Having dried these two discs, one is combined with the other on the motor shaft so that each color occupies half the circle. As soon as the motor starts, the two colors are no longer distinguished, and rapid rotation melts them so perfectly that the eye sees a new color, due to their mixture on the retina. This new color is a reddish gray, showing that the red is more chromatic than the blue-green. But by stopping the motor and sliding the green disc to cover more of the red one, there comes a point where rotation melts them into a perfectly neutral gray. No hint of either hue remains, and the pair is said to balance.

(109) Since this balance has been obtained by unequal areas of the two pigments, it must compensate for a lack of equal chroma in the hues (see paragraphs 76, 77); and, to measure this inequality, a slightly larger disc, with decimal divisions on its rim, is placed back of the two painted ones. If this scale shows the red as occupying 3⅓ parts of the area, while blue-green occupies 6⅔ parts, then the blue-green must be only half as chromatic as the red, since it takes twice as much to produce the balance.

(110) The red is then grayed (diminished in chroma by additions of a middle gray) until it can occupy half the circle, with blue-green on the remaining half, and still produce neutrality when mixed by rotation. Each disc now reads 5 on the decimal scale. Lest the graying of red should have disturbed its value, it is again tested on the photometric scale, and reads 4.7, showing it has been 69 slightly darkened by the graying process. A little white is therefore added until its value is restored to 5.

(111) The two opposites are now completely balanced, for they are equal in value (5), equal in chroma (5), and have proved their equality as complements by uniting in equal areas to form a neutral mixture. It only remains to apply them in their proper position on the sphere.

(112) A band is traced around the equator, divided in ten equal spaces, and lettered R, YR, Y, GY, G, BG, B, PB, P, and RP (see Fig. 18). This balanced red and blue-green are applied with the brush to spaces marked R and BG, care being taken to fill, but not to overstep the bounds, and the color laid absolutely flat, that no unevenness of value or chroma may disturb the balance.

(113) 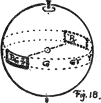The next pair, represented by Raw Sienna and Ultramarine, is similarly brought to middle value, balanced by equal areas on the Maxwell discs, and, when correct in each quality, is painted in the spaces Y and PB. Emerald Green and Purple Madder, which form the next pigment pair, are similarly tempered, proved, and applied, followed by the two remaining pairs, until the equator of the globe presents its ten equal steps of middle hues.

An equator of ten balanced hues.

(114) Now comes the total test of this circuit of balanced hues by rotation of the sphere. As it gains speed, the colors flash less and less, and finally melt into a middle gray of perfect neutrality. Had it failed to produce this gray and shown a tinge of any hue still persisting, we should say that the persistent hue was in excess, or, conversely, that its opposite hue was deficient in chroma, and failed to preserve its share in the balance.

70

(115) For instance, had rotation discovered the persistence of reddish gray, it would have proved the red too strong, or its opposite, blue-green, too weak, and we should have been forced to retrace our steps, applying a correction until neutrality was established by the rotation test.

(116) This is the practical demonstration of the assertion (Chapter I., paragraph 8) that a color has three dimensions which can be measured. Each of these ten middle hues has proved its right to a definite place on the color globe by its measurements of value and chroma. Being of equal chroma, all are equidistant from the neutral centre, and, being equal in value, all are equally removed from the poles. If the warm hues (red and yellow) or the cool hues (blue and green) were in excess, the rotation test of the sphere would fail to produce grayness, and so detect its lack of balance.29

A chromatic tuning fork.

(117) The five principal steps in this color equator are made in permanent enamel and carefully safeguarded, so that, if the pigments painted on the globe should change or become soiled, it could be at once detected and set right. These five are middle red (so called because midway between white and black, as well as midway between our strongest red and the neutral centre), middle yellow, middle green, middle blue, and middle purple. They may be called the CHROMATIC TUNING FORK, for they serve to establish the pitch of colors, as the musical tuning fork preserves the pitch of sounds.

Completion of a pigment color sphere.

(118) When the chromatic tuning fork has thus been obtained, 71 the completion of the globe is only a matter of patience, for the same method can be applied at any level in the scale of value, and a new circuit of balanced hues made to conform with its position between the poles of white and black.

(119) 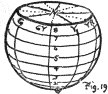The surface above and below the equatorial band is set off by parallels to match the photometric scale, making nine bands or value zones in all, of which the equator is fifth, the black pole being 0 and the white pole 10.

(120) Ten meridians carry the equatorial hues across all these value zones and trace the gradation of each hue through a complete scale from black to white, marked by their values, as shown in paragraph 68. Thus the red scale is R¹, R², R³, R⁴, R⁵ (middle red), R⁶, R⁷, R⁸, and R⁹, and similarly with each of the other hues. When the circle of hues corresponding to each level has been applied and tested, the entire surface of the globe is spread with a logical system of color scales, and the eye gratified with regular sequences which move by measured steps in each direction.

(121) Each meridian traces a scale of value for the hue in which it lies. Each parallel traces a scale of hue for the value at whose level it is drawn. Any oblique path across these scales traces a regular sequence, each step combining change of hue with a change of value and chroma. The more this path approaches the vertical, the less are its changes of hue and the more its changes of value and chroma; while, the nearer it comes to the horizontal, the less are its changes of value and chroma, while the greater become its changes of hue. Of these two oblique paths the first may be called that of a Luminist, or painter like Rembrandt, whose canvases present great contrasts of light and shade, while the second 72 is that of the Colorist, such as Titian, whose work shows great fulness of hues without the violent extremes of white and black.

Total balance of the sphere tested by rotation on any desired axis.

(122) Not only does the mount of the color sphere permit its rotation on the vertical axis (white-black), but it is so hung that it may be spun on the ends of any desired axis, as, for instance, that joining our first color pair, red and blue-green. With this pair as poles of rotation, a new equator is traced through all the values of purple on one side and of green-yellow on the other, which the rotation test melts in a perfect balance of middle gray, proving the correctness of these values. In the same way it may be hung and tested on successive axes, until the total balance of the entire spherical series is proved.

(123) But this color system does not cease with the colors spread on the surface of a globe.30 The first illustration of an orange filled with color was chosen for the purpose of stimulating the imagination to follow a surface color inward to the neutral axis by regular decrease of chroma. A slice at any level of the solid, as at value 8 (Fig. 10), shows each hue of that level passing by even steps of increasing grayness to the neutral gray N⁸ of the axis. In the case of red at this level, it is easily described by the notation R⁸/₃, R⁸/₂, R⁸/₁, of which the initial and upper numerals do not change, but the lower numeral traces loss of chroma by 3, 2, and 1 to the neutral axis.

(124) And there are stronger chromas of red outside the surface, which can be written R⁸/₄, R⁸/₅, R⁸/₆, etc. Indeed, our color measurements discover such differences of chroma in the various pigments used, that the color tree referred to in paragraphs 34, 35, is necessary 73 to bring before the eye their maximum chromas, most of which are well outside the spherical shell and at various levels of value. One way to describe the color sphere is to suggest that a color tree, the intervals between whose irregular branches are filled with appropriate color, can be placed in a turning lathe and turned down until the color maxima are removed, thus producing a color solid no larger than the chroma of its weakest pigment (Fig. 2).

Charts of the color solid.

(125) Thus it becomes evident that, while the color sphere is a valuable help to the child in conceiving color relations, in uniting the three scales of color measure, and in furnishing with its mount an excellent test of the theory of color balance, yet it is always restricted to the chroma of its weakest color, the surplus chromas of all other colors being thought of as enormous mountains built out at various levels to reach the maxima of our pigments.

(126) The complete color solid is, therefore, of irregular shape, with mountains and valleys, corresponding to the inequalities of pigments. To display these inequalities to the eye, we must prepare cross sections or charts of the solid, some horizontal, some vertical, and others oblique.

(127) Such a set of charts forms an atlas of the color solid, enabling one to see any color in its relation to all other colors, and name it by its degree of hue, value, and chroma. Fig. 20 is a horizontal chart of all colors which present middle value (5), and describes by an uneven contour the chroma of every hue at this level. The dotted fifth circle is the equator of the color sphere, whose principal hues, R⁵/₅. Y⁵/₅, G⁵/₅, B⁵/₅, and P⁵/₅, form the chromatic tuning fork, paragraph 117.

74

(128) In this single chart the eye readily distinguishes some three hundred different colors, each of which may be written by its hue, value, and chroma. And even the slightest variation of one of them can be defined. Thus, if the principal red were to fade slightly, so that it was a trifle lighter and a trifle weaker than the enamel, it would be written R^5.1/_4.9, showing it had lightened by 1 per cent. and weakened by 1 per cent. The discrimination made possible by this decimal notation is much finer than our present visual limit. Its use will stimulate finer perception of color.

(129) Such a very elementary sketch of the Color Solid and Color Atlas, which is all that can be given in the confines of this small book, will be elsewhere presented on a larger and more complete scale. It should be contrasted with the ideal form composed of prismatic colors, suggested in the last chapter, paragraphs 75 98, 99, which was shown to be impracticable, but whose ideal conditions it follows as far as the limitations of pigments permit.

(130) Besides its value in education as setting all our color notions in order, and supplying a simple method for their clear expression, it promises to do away with much of the misunderstanding that accompanies the every-day use of color.

(131) Popular color names are incongruous, irrational, and often ludicrous. One must smile in reading the list of 25 steps in a scale of blue, made by Schiffer-Muller in 1772:—

A.	a.	White pure.
	b.	White silvery or pearly.
	c.	White milky.
B.	a.	Bluish white.
	b.	Pearly white.
	c.	Watery white.
C.		Blue being born.
D.		Blue dying or pale.
E.		Mignon blue.
F.		Celestial blue, or sky-color.
G.	a.	Azure, or ultramarine.
	b.	Complete or perfect blue.
	c.	Fine or queen blue.
H.		Covert blue or turquoise.
I.		King blue (deep).
J.		Light brown blue or indigo.
K.	a.	Persian blue or woad flower.
	b.	Forge or steel blue.
	c.	Livid blue.
L.	a.	Blackish blue.
	b.	Hellish blue.
	c.	Black-blue.
M.	a.	Blue-black or charcoal.
	b.	Velvet black.
	c.	Jet black.

The advantage of spacing these 25 colors in 13 groups, some with three and others with but one example, is not apparent; nor why ultramarine should be several steps above turquoise, for the reverse is generally true. Besides which the hue of turquoise is greenish, while that of ultramarine is purplish, but the list cannot show this; and the remarkable statement that one kind of blue is “hellish,” while another is “celestial,” should rest upon an experience that few can claim. Failing to define color-value and color-hue, the list gives no hint of color-strength, except at 76 C and D, where one kind of blue is “dying” when the next is “being born,” which not inaptly describes the color memory of many a person. Finally, it assures us that Queen blue is “fine” and King blue is “deep.”

This year the fashionable shades are “burnt onion” and “fresh spinach.” The florists talk of a “pink violet” and a “green pink.” A maker of inks describes the red as a “true crimson scarlet,” which is a contradiction in terms. These and a host of other names borrowed from the most heterogeneous sources, become outlawed as soon as the simple color terms and measures of this system are adopted.

Color anarchy is replaced by systematic color description.

77

Appendix to Chapter V.

Color schemes based on Brewster’s mistaken theory.

Runge, of Hamburg (1810), suggested that red, yellow, and blue be placed equidistant around the equator of a sphere, with white and black at opposite poles. As the yellow was very light and the blue very dark, any coherency in the value scales of red, yellow, and blue was impossible.

Chevreul, of Paris (1861), seeking uniform color scales for his workmen at the Gobelins, devised a hollow cylinder built up of ten color circles. The upper circle had red, yellow, and blue spaced equidistant, and, as in Runge’s solid, yellow was very light and blue very dark. Each circle was then made “one-tenth” darker than the next above, until black was reached at the base. Although each circle was supposed to lie horizontally, only the black lowest circle presents a level of uniform values.

Yellow values increase their luminosity thrice as fast as purple values, so that each circle should tilt at an increasing angle, and the upper circle of strongest colors be inclined at 60° to the black base. Besides this fault shared with Runge’s sphere, it falls into another by not diminishing the size of the lower circles where added black diminishes the chroma.

Desire to make colors fit a chosen contour, and the absence of 78 measuring instruments, cause these schemes to ignore the facts of color relation. Like ancient maps made to satisfy a conqueror, they amuse by their distortion.

Brewster’s mistaken theory underlies these schemes, as is also the case with Froebel’s gifts, whose color balls continue to give wrong notions at the very threshold of color education. As pointed out in the Appendix to Chapter III., the “red-yellow-blue” theory inevitably spreads the warm field of yellow-red too far, and contracts the blue field, so that balance of color is rendered impossible, as illustrated in the gaudy chromo and flaming bill-board.

These schemes are criticised by Rood as “not only in the main arbitrary, but also vague”; and, although Chevreul’s charts were published by the government in most elaborate form, their usefulness is small. Interest in the growth of the present system, because of its measured character, led Professor Rood to give assistance in the tests, and at his request a color sphere was made for the Physical Cabinet at Columbia.

79

Chapter VI.
COLOR NOTATION.

Suggestion of a chromatic score.

(132) The last chapter traced a series of steps leading to the construction of a practical color sphere. Each color was tested by appropriate instruments to assure its degree of hue, value, and chroma, before being placed in position. Then the total sphere was tested to detect any lack of balance.

(133) Each color was also written by a letter and two numerals, showing its place in the three scales of hue, value, and chroma. This naturally suggests, not only a record of each separate color sensation, but also a union of these records in series and groups to form a color score, similar to the musical score by which the measured relations of sound are recorded.

(134) 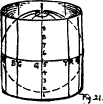A very simple form of color score may be easily imagined as a transparent envelope wrapped around the equator of the sphere, and forming a vertical cylinder (Fig. 21). On the envelope the equator traces a horizontal centre line, which is at 5 of the value scale, with zones 6, 7, 8, and 9 as parallels above, and the zones 4, 3, 2, and 1 below. Vertical lines are drawn through ten equidistant points on this centre line, corresponding with the divisions of the hue scale, and marked R, YR, Y, GY, G, BG, B, PB, P, and RP.

80

(135) The transparent envelope is thus divided into one hundred compartments, which provide for ten steps of value in each of the ten middle colors. Now, if we cut open this envelope along one of the verticals,—as, for instance, red-purple (RP), it may be spread out, making a flat chart of the color sphere (Fig. 22).

Why green is given the centre of the score.

(136) A cylindrical envelope might be opened on any desired meridian, but it is an advantage to have green (G) at the centre of the chart, and it is therefore opened at the opposite point, red-purple (RP). To the right of the green centre are the meridians of green-yellow (GY), yellow (Y), yellow-red (YR), and red (R), all of which are known as warm colors, because they contain yellow and red. To the left are the meridians of blue-green (BG), blue (B), purple-blue (PB), and purple (P), all of which are called cool colors, because they contain blue. Green, being neither warm nor cold of itself, and becoming so only by additions of yellow or of blue, thus serves as a balancing point or centre in the hue-scale.31

(137) The color score presents four large divisions or color fields made by the intersection of the equator with the meridian of green. Above the centre are all light colors, and below it are all dark colors. To the right of the centre are all warm colors, and to the left are all cool colors. Middle green (5G⁵/₅) is the centre of balance for these contrasted qualities, recognized by all 81 practical color workers. The chart forms a rectangle whose length equals the equator of the color sphere and its height equals the axis (a proportion of 3.14:1), representing a union and balance of the scales of hue and of value. This provides for two color dimensions; but, to be complete, the chart must provide for the third dimension, chroma.

(138) Replacing the chart around the sphere and joining its ends, so that it re-forms the transparent envelope, we may thrust a pin through at any point until it pierces the surface of the sphere. Indeed, the pin can be thrust deeper until it reaches the neutral axis, thus forming a scale of chroma for the color point where it enters (see paragraph 12). In the same way any colors on the sphere, within the sphere, or without it, can have pins thrust into the chart to mark their place, and the length by which each pin projects can be taken as a measure of chroma. If the chart is now unrolled, it retains the pins, which by their place describe the hue and value of a color, while their length describes its chroma.

Pins stuck into the score represent chroma.

(139) With this idea of the third color dimension incorporated in the score we can discard the pin, and record its length by a numeral. Any dot placed on the score marks a certain degree of hue and value, while a numeral beside it marks the degree of chroma which it carries, uniting with the hue and value of that point to give us a certain color. Glancing over a series of such color points, the eye easily grasps their individual character, and connects them into an intelligible series.

(140) Thus a flat chart becomes the projection of the color solid, and any color in that solid is transferred to the surface of the chart, retaining its degrees of hue, value, and chroma. So far the scales have been spoken of as divided into ten steps, but 82 they may be subdivided much finer, if desired, by use of the decimal point. It is a question of convenience whether to make a small score with only the large divisions, or a much larger score with a hundred times as many steps. In the latter case each hue has ten steps, the middle step of green being distinguished as 5G⁵/₅ to suggest the four steps 1G, 2G, 3G, 4G, which precede it, and 6G, 7G, 8G, and 9G, which follow it toward blue-green.

The score preserves color records in a convenient shape.

Such a color score, or notation diagram, to be made small or large as the case demands, offers a very convenient means for recording color combinations, when pigments are not at hand.

(141) 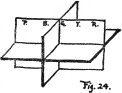To display its three dimensions, a little model can be made with three visiting cards, so placed as to present their mutual intersection at right angles (Fig. 24).

5G ⁵/₅ is their centre of mutual balance. A central plane separates all colors into two contrasted fields. To the right are all warm colors, to the left are all cool colors. Each of these 83 fields is again divided by the plane of the equator into lighter colors above and darker colors below. These four color fields are again subdivided by a transverse plane through 5G⁵/₅ into strong colors in front and weak colors beyond or behind it.

(142) Any color group, whose record must all be written to the right of the centre, is warm, because red and yellow are dominant. One to the left of the centre must be cool, because it is dominated by blue. A group written all above the centre must have light in excess, while one written entirely below is dark to excess. Finally, a score written all in front of the centre represents only strong chromas, while one written behind it contains only weak chromas. From this we gather that a balanced composition of color preserves some sort of equilibrium, uniting degrees of warm and cool, of light and dark, and of weak and strong, which is made at once apparent by the dots on the score.

(143) A single color, like that of a violet, a rose, or a buttercup, appears as a dot on the score, with a numeral added for its chroma. A parti-colored flower, such as a nasturtium, is shown by two dots with their chromas, and a bunch of red and yellow flowers will give by their dots a color passage, or “silhouette,” whose warmth and lightness is unmistakable.

The chroma of each flower written with the silhouette completes the record. The hues of a beautiful Persian rug, with dark red predominating, or a verdure tapestry, in which green is dominant, or a Japanese print, with blue dominant, will trace upon the score a pattern descriptive of its color qualities. These records, with practice, become as significant to the eye as the musical score. The general character of a color combination is apparent at a glance, while its degrees of chroma are readily joined to fill out the mental image.

84

(144) Such a plan of color notation grows naturally from the spherical system of measured colors. It is hardly to be hoped, in devising a color score, that it should not seem crude at first. But the measures forming the basis of this record can be verified by impartial instruments, and have a permanent value in the general study of color. They also afford some definite data as to personal bias in color estimates.

(145) This makes it possible to collect in a convenient form two contrasting and valuable records, one preserving such effects of color as are generally called pleasing, and another of such groups as are found unpleasant to the eye. Out of such material something may be gained, more reliable than the shifting, personal, and contradictory statements about color harmony now prevalent.

31. To put this in terms of the spectrum wave lengths, long waves at the red end of the spectrum give the sensation of warmth, while short waves at the violet end cause the sensation of coolness. Midway between these extremes is the wave length of green.

85

Chapter VII.
COLOR HARMONY.

Colors may be grouped to please or to give annoyance.

(146) Attempts to define the laws of harmonious color have not attained marked success, and the cause is not far to seek. The very sensations underlying these effects of concord or of discord are themselves undefined. The misleading formula of my student days—that three parts of yellow, five parts of red, and eight parts of blue would combine harmoniously—was unable to define the kind of red, yellow, and blue intended; that is, the hue, value, and chroma of each of these colors was unknown, and the formula meant a different thing to each person who tried to use it.

(147) It is true that a certain red, green, and blue can be united in such proportions on Maxwell discs as to balance in a neutral gray; but the slightest change in either the hue, value, or chroma, of any one of them, upsets the balance. A new proportion is then needed to regain the neutral mixture. This has already been shown in the discussion of triple balance (paragraph 82).

(148) Harmony of color has been still further complicated by the use of terms that belong to musical harmony. Now music is a measured art, and has found a set of intervals which are defined scientifically. The two arts have many points of similarity; and the impulses of sound waves on the ear, like those of light waves on the eye, are measured vibrations. But they are far apart in their scales, and differ so much in important 86 particulars that no practical relationship can be set up. The intervals of color sensation require fit names and measures, ere their infinite variety can be organized into a fixed system.

(149) Any effort to compare certain sounds to certain colors soon leads to the wildest vagaries.

Harmony of sound is unlike harmony of color.

(150) The poverty of color language tempts to a borrowing from the richer terminology of music. Musical terms, such as “pitch, key, note, tone, chord, modulation, nocturne, and symphony,” are frequently used in the description of color, serving by association to convey certain vague ideas.

(151) In the same way the term color harmony, from association with musical harmony, presents to the mind an image of color arrangement,—varied, yet well proportioned, grouped in orderly fashion, and agreeable to the eye. But any attempt to define this image in terms of color is disappointing. Here is a beautiful Persian rug: why do we call it beautiful? One says “because its colors are rich.” Why are they rich? “Because they are deep in tone.” What does that mean? The double-bass and the fog-horn are deep in tone, but not necessarily beautiful on that account. “Oh, no,” says another, “it is all in one harmonious key.” But what is a key of color? Is it made by all the values of one color, such as red, or by all the hues of equal value, such as the middle hues in our color solid?

(152) Certainly it is neither, for the rug has both light and dark colors; and, of the reds, yellows, greens, and blues, some are stronger and others weaker. Then what do we mean by a key of color? One must either continue to flounder about or frankly confess ignorance.

(153) Musical harmony explains itself in clear language. It 87 is illustrated by fixed and definite sound intervals, whose measured relations form the basis of musical composition. Each key has an unmistakable character, and the written score presents a statement that means practically the same thing to every person of musical intelligence. But the adequate terms of color harmony are yet to be worked out.

Let us leave these musical analogies, retaining only the clue that a measured and orderly relation underlies the idea of harmony. The color solid which has been the subject of these pages is built upon measured color relations. It unites measured scales of hue, value, and chroma, and gives a definite color name to every sensation from the maxima of color-light and color-strength to their disappearance in darkness.

(154) Must not this theoretical color solid, therefore, locate all the elements which combine to produce color harmony or color discord?32

(155) Instead of theorizing, let us experiment. As a child at the piano, who first strikes random and widely separated notes, but soon seeks for the intervals of a familiar air, so let us, after roaming over the color globe and its charts, select one familiar color, and study what others will combine with it to please the eye.

(156) Here is a grayish green stuff for a dress, and the little girl who is to wear it asks what other colors she may use with it. First let us find it on our instrument, so as to realize its relation to other degrees of color. Its value is 6,—one step above the equator of middle value. Its hue is green, G, and its chroma 5. It is written G⁶/₅.

(157) Color paths lead out from this point in every direction. 88 Where shall we find harmonious colors, where discordant, where those paths most frequently travelled? Are there new ones still to be explored?

(158) There are three typical paths: one vertical, with rapid change of value; another lateral, with rapid change of hue; and a third inward, through the neutral centre to seek the opposite color field. All other paths are combinations of two or three of these typical directions in the color solid.

Three typical color paths.

(159) 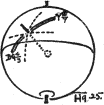1. The vertical path finds only lighter and darker values of gray-green,—“self-colors or shades,” they are generally called,—and offers a safe path, even for those deficient in color sensation, avoiding all complications of hue, and leaving the eye free to estimate different degrees of a single quality,—color-light.

(160) 2. The lateral path passes through neighboring hues on either side. In this case it is a sequence from blue, through green into yellow. This is simply change of hue, without change of value or chroma if the path be level, but, by inclining it, one end of the sequence becomes lighter, while the other end darkens. It thus becomes an intermediate between the first and second typical paths, combining, at each step, a change of hue with a change of value. This is more complicated, but also more interesting, showing how the character of the gray-green dress will be set off by a lighter hat of Leghorn straw, and further improved by a trimming of darker blue-green. The sequence can be made still more subtle and attractive by choosing a straw whose yellow is stronger than the green of the dress, while a weaker 89 chroma of blue-green is used in the trimming. This is clearly expressed by the notation thus: Y⁸/₇, G⁶/₅, BG⁴/₃, and written on the score by three dots and their chromas,—7, 5, and 3 (see Fig. 23).

(161) 3. The inward path which leads by increase of gray to the neutral centre, and on to the opposite hue red-purple, RP⁴/₅, is full of pitfalls for the inexpert. It combines great change of hue and chroma, with small change of value.

(162) If any other color point be chosen in place of gray-green, the same typical paths are just as easily traced, written by the notation, and recorded on the color score.

These paths trace sequences from any point in the color solid.

(163) In the construction of the color solid we saw that its scales were made of equal steps in hue, value, and chroma, and tested by balance on the centre of neutral gray. Any step will serve as a point of departure to trace regular sequences of the three types. The vertical type is a sequence of value only. It is somewhat tame, lacking the change of hue and chroma, but giving a monotonous harmony of regular values. The horizontal type traces a sequence of neighboring hues, less tame than the vertical type, but monotonous in value and chroma. The inward type connects opposite hues by a sequence of chroma balanced on middle gray, and is more stimulating to the eyes.

(164) These paths have so far been treated as made up of equal steps in each direction, with the accompanying idea of equal quantities of color at each step. But by using unequal quantities of color, the balance may be preserved by compensations to the intervals that separate the colors (see paragraphs 109, 110).

Unequal color quantities compensated by relations of hue, value, and chroma.

(165) Small bits of powerful color can be used to balance large 90 fields of weak chroma. For instance, a spot of strong reddish purple is balanced and enhanced by a field of gray-green. So an amethyst pin at the neck of the girl’s dress will appear to advantage with the gown, and also with the Leghorn straw. But a large field of strong color, such as a cloth jacket of reddish purple, would be fatal to the measured harmony we seek.

(166) This use of a small point of strong chroma, if repeated at intervals, sets up a notion of rhythm; but, in order to be rhythmic, there must be recurrent emphasis, “a succession of similar units, combining unlike elements.” This quality must not be confused with the unaccented succession, seen in a measured scale of hue, value, or chroma.

Paper masks to isolate color intervals.

(167) A sheet of paper large enough to hide the color sphere may be perforated with three or more openings in a straight line, and applied against the surface, so as to isolate the steps of any sequence which we wish to study. Thus the sequence given in paragraph 160—Y⁸/₇, G⁶/₅, BG⁴/₃—may be changed to bring it on the surface of the sphere, when it reads Y⁸/₃, G⁶/₅, BG⁵/₅. A mask with round holes, spaced so as to uncover these three spots, relieves the eye from the distraction of other colors. Keeping the centre spot on green, the mask may be moved so as to study the effect of changing hue or value of the other two steps in the sequence.

(168) The sequence is lightened by sliding the whole mask upward, and darkened by dropping it lower. Then the result of using the same intervals in another field is easily studied by moving the mask to another part of the solid.

(169) Change of interval immediately modifies the character of a color sequence. This is readily shown by having an under-mask, with a long, continuous slit, and an over-mask whose perforations 91 are arranged in several rows, each row giving different spaces between the perforations. In the case of the girl’s clothing, the same sequence produces quite a different effect, if two perforations of the over-mask are brought nearer to select a lighter yellow-green dress, while the ends of the sequence remain unchanged. To move the middle perforation near the other end, selects a darker bluish green dress, on which the trimming will be less contrasted, while the hat appears brighter than before, because of greater contrast.

(170) The variations of color sequence which can thus be studied out by simple masks are almost endless; yet upon a measured system the character of each effect is easily described, and, if need be, preserved by a written record.

Invention of color groups.

(171) Experiments with variable masks for the selection of color intervals, such as have been described, soon stimulate the imagination, so that it conceives sequences through any part of the color solid. The color image becomes a permanent mental adjunct. Five middle colors, tempered with white and black, permit us to devise the greatest variety of sequences, some light, others dark, some combining small difference of chroma with large difference of hue, others uniting large intervals of chroma with small intervals of hue, and so on through a well-nigh inexhaustible series.

(172) As this constructive imagination gains power, the solid and its charts may be laid aside. We can now think color consecutively. Each color suggests its place in the system, and may be taken as a point of departure for the invention of groups to carry out a desired relation.

(173) This selective mental process is helped by the score described 92 in the last chapter; and the quantity of each color chosen for the group is easily indicated by a variable circle, drawn round the various points on the diagram. Thus, in the case of the child’s clothes, a large circle around G⁶/₅ gives the area of that color as compared with smaller circles around Y⁸/₇ and BG⁴/₃, representing the area of the straw and the trimming.

(174) When the plotting of color groups has become instinctive from long practice, it opens a wide field of color study. Take as illustration the wings of butterflies or the many varieties of pansies. These fascinating color schemes can be written with indications of area that record their differences by a simple diagram. In the same way, rugs, tapestries, mosaics,—whatever attracts by its beauty and harmony of color,—can be recorded and studied in measured terms; and the mental process of estimating hues, values, chromas, and areas by established scales must lead the color sense to finer and finer perceptions.

The same process serves as well to record the most annoying and inharmonious color groups. When sufficient of these records have been obtained, they furnish definite material for a contrast of the color combinations which please, with those that cause disgust. Such a contrast should discover some broad law of color harmony. It will then be in measured terms which can be clearly given; not a vague personal statement, conveying different meanings to each one who hears it.

Constant exercise needed to train the color sense.

(175) Appreciation of beautiful color grows by exercise and discrimination, just as naturally as fine perception of music or literature. Each is an outlet for the expression of taste,—a language which may be used clumsily or with skill.

(176) As color perception becomes finer, it discards the more 93 crude and violent contrasts. A child revels in strong chromas, but the mark of a colorist is ability to employ low chroma without impoverishing the color effect. As a boy’s shrieks and groans can be tempered to musical utterance, so his debauches in violent red, green, and purple must be replaced by tempered hues.

(177) Raphael, Titian, Velasquez, Corot, Chavannes, and Whistler are masters in the use of gray. Personal bias may lead one colorist a little more toward warm colors, and another slightly toward the cool field, in each case attaining a sense of harmonious balance by tempered degrees of value and chroma.33

(178) It is not claimed that discipline in the use of subtle colors will make another Corot or Velasquez, but it will make for comprehension of their skill. It is grotesque to watch gaudily dressed persons going into ecstasies over the delicate coloring of a Botticelli, when the internal as well as the external evidence is against them.

(179) The colors which we choose, not only in personal apparel, but in our rooms and decorations, are mute witnesses to a stage of color perception.

If that perception is trained to finer distinctions, the mind can no longer be content with coarse expression. It begins to feel an incongruity between the “loud” color of the wall paper, bought because it was fashionable, and the quiet hues of the rug, which was a gift from some artistic friend. It sees that, although the furniture is covered with durable and costly materials, their color “swears” at that of the curtains and wood-work. In short, the 94 room has been jumbled together at various periods, without any plan or sense of color design.

(180) Good taste demands that a room be furnished, not alone for convenience and comfort, but also with an eye to the beauty of the various objects, so that, instead of confusing and destroying the colors, each may enhance the other. And, when this sense of color harmony is aroused, it selects and arranges the books, the rugs, the lamp shade, the souvenirs of travel and friendship, the wall paper, pictures, and hangings, so that they fit into a color scheme, not only charming to the eye at first glance, but which continues to please the mind as it traces out an intelligent plan, bringing all into general harmony.

(181) Nor will this cease when one room has been put to rights. Such a coloristic attitude is not satisfied until the vista into the next apartment is made attractive. Or should there be a suite of rooms, it demands that, with variety in each one, they all be brought into harmonious sequence. Thus the study of color finds immediate and practical use in daily life. It is a needed discipline of color vision, in the sense that geometry is a discipline of the mind, and it also enters into the pleasure and refinement of life at every step. Skill or awkwardness in its use exerts as positive an influence upon us as do the harmonies and discords of sound, and a far more continuous one. It is thought a defect to be unmusical. Should it not be considered a mark of defective cultivation to be insensitive to color?

(182) In this slight sketch of color education it has been assumed that we are to deal with those who have normal perceptions. But there are some who inherit or develop various degrees of color-blindness; and a word in their behalf may be opportune.

(183) A case of total color-blindness is very rare, but a few 95 are on record. When a child shows deficient color perception,34 a little care may save him much discomfort, and patient training may correct it. If he mismatches some hues, confuses their names, seems incapable of the finer distinctions of color, study to find the hues which he estimates well, and then help him to venture a little into that field where his perception is at fault. Improvement is pretty sure to follow when this is sympathetically done. One student, who never outgrew the habit of giving a purplish hue to all his work, despite many expedients and the use of various lights and colored objects to correct it, is the single exception among hundreds whom it has been my privilege to watch as they improved their first crude estimates, and gained skill in expressing their sense of Nature’s subtle color.

(184) To sum up, the first chapter suggests a measured color system in place of guess-work. The next describes the three color qualities, and sketches a child’s growth in color perception. The third tells how colors may be mingled in such proportions as to balance. After the impracticability of using spectral color has been shown in the fourth chapter, the fifth proceeds to build a practical color solid. The sixth provides for a written record of color, and the last applies all that has preceded to suggestions for the study of color harmony.

(185) Wide gaps appear in this outline. There is much that deserves fuller treatment. But, if the search for refined color and a clearer outlook upon its relations are stimulated by this fragmentary sketch, some of its faults may be overlooked.

 
REPRODUCTION OF FLOWER STUDIES,
PAINTED WITH MUNSELL WATER COLOR
Published by
Wadsworth, Howland & Co., Incorporated, Boston, Mass.

End of the Project Gutenberg EBook of A Color Notation, by Albert H. Munsell

*** END OF THIS PROJECT GUTENBERG EBOOK A COLOR NOTATION ***

***** This file should be named 26054-h.htm or 26054-h.zip *****
This and all associated files of various formats will be found in:
        http://www.gutenberg.org/2/6/0/5/26054/

Produced by Louise Hope, K.D. Thornton and the Online
Distributed Proofreading Team at http://www.pgdp.net


Updated editions will replace the previous one--the old editions
will be renamed.

Creating the works from public domain print editions means that no
one owns a United States copyright in these works, so the Foundation
(and you!) can copy and distribute it in the United States without
permission and without paying copyright royalties.  Special rules,
set forth in the General Terms of Use part of this license, apply to
copying and distributing Project Gutenberg-tm electronic works to
protect the PROJECT GUTENBERG-tm concept and trademark.  Project
Gutenberg is a registered trademark, and may not be used if you
charge for the eBooks, unless you receive specific permission.  If you
do not charge anything for copies of this eBook, complying with the
rules is very easy.  You may use this eBook for nearly any purpose
such as creation of derivative works, reports, performances and
research.  They may be modified and printed and given away--you may do
practically ANYTHING with public domain eBooks.  Redistribution is
subject to the trademark license, especially commercial
redistribution.



*** START: FULL LICENSE ***

THE FULL PROJECT GUTENBERG LICENSE
PLEASE READ THIS BEFORE YOU DISTRIBUTE OR USE THIS WORK

To protect the Project Gutenberg-tm mission of promoting the free
distribution of electronic works, by using or distributing this work
(or any other work associated in any way with the phrase "Project
Gutenberg"), you agree to comply with all the terms of the Full Project
Gutenberg-tm License (available with this file or online at
http://gutenberg.org/license).


Section 1.  General Terms of Use and Redistributing Project Gutenberg-tm
electronic works

1.A.  By reading or using any part of this Project Gutenberg-tm
electronic work, you indicate that you have read, understand, agree to
and accept all the terms of this license and intellectual property
(trademark/copyright) agreement.  If you do not agree to abide by all
the terms of this agreement, you must cease using and return or destroy
all copies of Project Gutenberg-tm electronic works in your possession.
If you paid a fee for obtaining a copy of or access to a Project
Gutenberg-tm electronic work and you do not agree to be bound by the
terms of this agreement, you may obtain a refund from the person or
entity to whom you paid the fee as set forth in paragraph 1.E.8.

1.B.  "Project Gutenberg" is a registered trademark.  It may only be
used on or associated in any way with an electronic work by people who
agree to be bound by the terms of this agreement.  There are a few
things that you can do with most Project Gutenberg-tm electronic works
even without complying with the full terms of this agreement.  See
paragraph 1.C below.  There are a lot of things you can do with Project
Gutenberg-tm electronic works if you follow the terms of this agreement
and help preserve free future access to Project Gutenberg-tm electronic
works.  See paragraph 1.E below.

1.C.  The Project Gutenberg Literary Archive Foundation ("the Foundation"
or PGLAF), owns a compilation copyright in the collection of Project
Gutenberg-tm electronic works.  Nearly all the individual works in the
collection are in the public domain in the United States.  If an
individual work is in the public domain in the United States and you are
located in the United States, we do not claim a right to prevent you from
copying, distributing, performing, displaying or creating derivative
works based on the work as long as all references to Project Gutenberg
are removed.  Of course, we hope that you will support the Project
Gutenberg-tm mission of promoting free access to electronic works by
freely sharing Project Gutenberg-tm works in compliance with the terms of
this agreement for keeping the Project Gutenberg-tm name associated with
the work.  You can easily comply with the terms of this agreement by
keeping this work in the same format with its attached full Project
Gutenberg-tm License when you share it without charge with others.

1.D.  The copyright laws of the place where you are located also govern
what you can do with this work.  Copyright laws in most countries are in
a constant state of change.  If you are outside the United States, check
the laws of your country in addition to the terms of this agreement
before downloading, copying, displaying, performing, distributing or
creating derivative works based on this work or any other Project
Gutenberg-tm work.  The Foundation makes no representations concerning
the copyright status of any work in any country outside the United
States.

1.E.  Unless you have removed all references to Project Gutenberg:

1.E.1.  The following sentence, with active links to, or other immediate
access to, the full Project Gutenberg-tm License must appear prominently
whenever any copy of a Project Gutenberg-tm work (any work on which the
phrase "Project Gutenberg" appears, or with which the phrase "Project
Gutenberg" is associated) is accessed, displayed, performed, viewed,
copied or distributed:

This eBook is for the use of anyone anywhere at no cost and with
almost no restrictions whatsoever.  You may copy it, give it away or
re-use it under the terms of the Project Gutenberg License included
with this eBook or online at www.gutenberg.org

1.E.2.  If an individual Project Gutenberg-tm electronic work is derived
from the public domain (does not contain a notice indicating that it is
posted with permission of the copyright holder), the work can be copied
and distributed to anyone in the United States without paying any fees
or charges.  If you are redistributing or providing access to a work
with the phrase "Project Gutenberg" associated with or appearing on the
work, you must comply either with the requirements of paragraphs 1.E.1
through 1.E.7 or obtain permission for the use of the work and the
Project Gutenberg-tm trademark as set forth in paragraphs 1.E.8 or
1.E.9.

1.E.3.  If an individual Project Gutenberg-tm electronic work is posted
with the permission of the copyright holder, your use and distribution
must comply with both paragraphs 1.E.1 through 1.E.7 and any additional
terms imposed by the copyright holder.  Additional terms will be linked
to the Project Gutenberg-tm License for all works posted with the
permission of the copyright holder found at the beginning of this work.

1.E.4.  Do not unlink or detach or remove the full Project Gutenberg-tm
License terms from this work, or any files containing a part of this
work or any other work associated with Project Gutenberg-tm.

1.E.5.  Do not copy, display, perform, distribute or redistribute this
electronic work, or any part of this electronic work, without
prominently displaying the sentence set forth in paragraph 1.E.1 with
active links or immediate access to the full terms of the Project
Gutenberg-tm License.

1.E.6.  You may convert to and distribute this work in any binary,
compressed, marked up, nonproprietary or proprietary form, including any
word processing or hypertext form.  However, if you provide access to or
distribute copies of a Project Gutenberg-tm work in a format other than
"Plain Vanilla ASCII" or other format used in the official version
posted on the official Project Gutenberg-tm web site (www.gutenberg.org),
you must, at no additional cost, fee or expense to the user, provide a
copy, a means of exporting a copy, or a means of obtaining a copy upon
request, of the work in its original "Plain Vanilla ASCII" or other
form.  Any alternate format must include the full Project Gutenberg-tm
License as specified in paragraph 1.E.1.

1.E.7.  Do not charge a fee for access to, viewing, displaying,
performing, copying or distributing any Project Gutenberg-tm works
unless you comply with paragraph 1.E.8 or 1.E.9.

1.E.8.  You may charge a reasonable fee for copies of or providing
access to or distributing Project Gutenberg-tm electronic works provided
that

- You pay a royalty fee of 20% of the gross profits you derive from
     the use of Project Gutenberg-tm works calculated using the method
     you already use to calculate your applicable taxes.  The fee is
     owed to the owner of the Project Gutenberg-tm trademark, but he
     has agreed to donate royalties under this paragraph to the
     Project Gutenberg Literary Archive Foundation.  Royalty payments
     must be paid within 60 days following each date on which you
     prepare (or are legally required to prepare) your periodic tax
     returns.  Royalty payments should be clearly marked as such and
     sent to the Project Gutenberg Literary Archive Foundation at the
     address specified in Section 4, "Information about donations to
     the Project Gutenberg Literary Archive Foundation."

- You provide a full refund of any money paid by a user who notifies
     you in writing (or by e-mail) within 30 days of receipt that s/he
     does not agree to the terms of the full Project Gutenberg-tm
     License.  You must require such a user to return or
     destroy all copies of the works possessed in a physical medium
     and discontinue all use of and all access to other copies of
     Project Gutenberg-tm works.

- You provide, in accordance with paragraph 1.F.3, a full refund of any
     money paid for a work or a replacement copy, if a defect in the
     electronic work is discovered and reported to you within 90 days
     of receipt of the work.

- You comply with all other terms of this agreement for free
     distribution of Project Gutenberg-tm works.

1.E.9.  If you wish to charge a fee or distribute a Project Gutenberg-tm
electronic work or group of works on different terms than are set
forth in this agreement, you must obtain permission in writing from
both the Project Gutenberg Literary Archive Foundation and Michael
Hart, the owner of the Project Gutenberg-tm trademark.  Contact the
Foundation as set forth in Section 3 below.

1.F.

1.F.1.  Project Gutenberg volunteers and employees expend considerable
effort to identify, do copyright research on, transcribe and proofread
public domain works in creating the Project Gutenberg-tm
collection.  Despite these efforts, Project Gutenberg-tm electronic
works, and the medium on which they may be stored, may contain
"Defects," such as, but not limited to, incomplete, inaccurate or
corrupt data, transcription errors, a copyright or other intellectual
property infringement, a defective or damaged disk or other medium, a
computer virus, or computer codes that damage or cannot be read by
your equipment.

1.F.2.  LIMITED WARRANTY, DISCLAIMER OF DAMAGES - Except for the "Right
of Replacement or Refund" described in paragraph 1.F.3, the Project
Gutenberg Literary Archive Foundation, the owner of the Project
Gutenberg-tm trademark, and any other party distributing a Project
Gutenberg-tm electronic work under this agreement, disclaim all
liability to you for damages, costs and expenses, including legal
fees.  YOU AGREE THAT YOU HAVE NO REMEDIES FOR NEGLIGENCE, STRICT
LIABILITY, BREACH OF WARRANTY OR BREACH OF CONTRACT EXCEPT THOSE
PROVIDED IN PARAGRAPH F3.  YOU AGREE THAT THE FOUNDATION, THE
TRADEMARK OWNER, AND ANY DISTRIBUTOR UNDER THIS AGREEMENT WILL NOT BE
LIABLE TO YOU FOR ACTUAL, DIRECT, INDIRECT, CONSEQUENTIAL, PUNITIVE OR
INCIDENTAL DAMAGES EVEN IF YOU GIVE NOTICE OF THE POSSIBILITY OF SUCH
DAMAGE.

1.F.3.  LIMITED RIGHT OF REPLACEMENT OR REFUND - If you discover a
defect in this electronic work within 90 days of receiving it, you can
receive a refund of the money (if any) you paid for it by sending a
written explanation to the person you received the work from.  If you
received the work on a physical medium, you must return the medium with
your written explanation.  The person or entity that provided you with
the defective work may elect to provide a replacement copy in lieu of a
refund.  If you received the work electronically, the person or entity
providing it to you may choose to give you a second opportunity to
receive the work electronically in lieu of a refund.  If the second copy
is also defective, you may demand a refund in writing without further
opportunities to fix the problem.

1.F.4.  Except for the limited right of replacement or refund set forth
in paragraph 1.F.3, this work is provided to you 'AS-IS' WITH NO OTHER
WARRANTIES OF ANY KIND, EXPRESS OR IMPLIED, INCLUDING BUT NOT LIMITED TO
WARRANTIES OF MERCHANTIBILITY OR FITNESS FOR ANY PURPOSE.

1.F.5.  Some states do not allow disclaimers of certain implied
warranties or the exclusion or limitation of certain types of damages.
If any disclaimer or limitation set forth in this agreement violates the
law of the state applicable to this agreement, the agreement shall be
interpreted to make the maximum disclaimer or limitation permitted by
the applicable state law.  The invalidity or unenforceability of any
provision of this agreement shall not void the remaining provisions.

1.F.6.  INDEMNITY - You agree to indemnify and hold the Foundation, the
trademark owner, any agent or employee of the Foundation, anyone
providing copies of Project Gutenberg-tm electronic works in accordance
with this agreement, and any volunteers associated with the production,
promotion and distribution of Project Gutenberg-tm electronic works,
harmless from all liability, costs and expenses, including legal fees,
that arise directly or indirectly from any of the following which you do
or cause to occur: (a) distribution of this or any Project Gutenberg-tm
work, (b) alteration, modification, or additions or deletions to any
Project Gutenberg-tm work, and (c) any Defect you cause.


Section  2.  Information about the Mission of Project Gutenberg-tm

Project Gutenberg-tm is synonymous with the free distribution of
electronic works in formats readable by the widest variety of computers
including obsolete, old, middle-aged and new computers.  It exists
because of the efforts of hundreds of volunteers and donations from
people in all walks of life.

Volunteers and financial support to provide volunteers with the
assistance they need, is critical to reaching Project Gutenberg-tm's
goals and ensuring that the Project Gutenberg-tm collection will
remain freely available for generations to come.  In 2001, the Project
Gutenberg Literary Archive Foundation was created to provide a secure
and permanent future for Project Gutenberg-tm and future generations.
To learn more about the Project Gutenberg Literary Archive Foundation
and how your efforts and donations can help, see Sections 3 and 4
and the Foundation web page at http://www.pglaf.org.


Section 3.  Information about the Project Gutenberg Literary Archive
Foundation

The Project Gutenberg Literary Archive Foundation is a non profit
501(c)(3) educational corporation organized under the laws of the
state of Mississippi and granted tax exempt status by the Internal
Revenue Service.  The Foundation's EIN or federal tax identification
number is 64-6221541.  Its 501(c)(3) letter is posted at
http://pglaf.org/fundraising.  Contributions to the Project Gutenberg
Literary Archive Foundation are tax deductible to the full extent
permitted by U.S. federal laws and your state's laws.

The Foundation's principal office is located at 4557 Melan Dr. S.
Fairbanks, AK, 99712., but its volunteers and employees are scattered
throughout numerous locations.  Its business office is located at
809 North 1500 West, Salt Lake City, UT 84116, (801) 596-1887, email
business@pglaf.org.  Email contact links and up to date contact
information can be found at the Foundation's web site and official
page at http://pglaf.org

For additional contact information:
     Dr. Gregory B. Newby
     Chief Executive and Director
     gbnewby@pglaf.org


Section 4.  Information about Donations to the Project Gutenberg
Literary Archive Foundation

Project Gutenberg-tm depends upon and cannot survive without wide
spread public support and donations to carry out its mission of
increasing the number of public domain and licensed works that can be
freely distributed in machine readable form accessible by the widest
array of equipment including outdated equipment.  Many small donations
($1 to $5,000) are particularly important to maintaining tax exempt
status with the IRS.

The Foundation is committed to complying with the laws regulating
charities and charitable donations in all 50 states of the United
States.  Compliance requirements are not uniform and it takes a
considerable effort, much paperwork and many fees to meet and keep up
with these requirements.  We do not solicit donations in locations
where we have not received written confirmation of compliance.  To
SEND DONATIONS or determine the status of compliance for any
particular state visit http://pglaf.org

While we cannot and do not solicit contributions from states where we
have not met the solicitation requirements, we know of no prohibition
against accepting unsolicited donations from donors in such states who
approach us with offers to donate.

International donations are gratefully accepted, but we cannot make
any statements concerning tax treatment of donations received from
outside the United States.  U.S. laws alone swamp our small staff.

Please check the Project Gutenberg Web pages for current donation
methods and addresses.  Donations are accepted in a number of other
ways including checks, online payments and credit card donations.
To donate, please visit: http://pglaf.org/donate


Section 5.  General Information About Project Gutenberg-tm electronic
works.

Professor Michael S. Hart is the originator of the Project Gutenberg-tm
concept of a library of electronic works that could be freely shared
with anyone.  For thirty years, he produced and distributed Project
Gutenberg-tm eBooks with only a loose network of volunteer support.


Project Gutenberg-tm eBooks are often created from several printed
editions, all of which are confirmed as Public Domain in the U.S.
unless a copyright notice is included.  Thus, we do not necessarily
keep eBooks in compliance with any particular paper edition.


Most people start at our Web site which has the main PG search facility:

     http://www.gutenberg.org

This Web site includes information about Project Gutenberg-tm,
including how to make donations to the Project Gutenberg Literary
Archive Foundation, how to help produce our new eBooks, and how to
subscribe to our email newsletter to hear about new eBooks.